Absolute Value
The number's distance from 0 on a number line. The absolute value of a number
x is denoted by |
x|, and those bars always make what's inside turn positive:
|
x| =
x|
-x| =
xSo with absolute value, we're not concerned with whether
x is positive or negative, we just want to know how far it is away from 0. Because 0 has had some problems with
x in the past, and has applied for a restraining order.
The value of a number when you strip it naked of any negative signs. It's also the distance the number is away from zero on the number line.
The distance a number is from 0. The absolute value is always positive (or 0 if we have | 0 |).
Two bars that drop around an expression and force everything to be sunshine and rainbows: real positive stuff.
The magnitude of a number when sign is not considered. Or, the positive version of the story. It's like when you tell your friends you might have failed the driver's test, but at least you got some parallel parking practice out of it.
Any negative value inside an absolute value sign is changed to positive.
The magnitude of a number, irrespective of sign. It works great when we talk about distance, but not so great when we talk about temperature.
Ok, ok. You know the mathy absolute value thing from like 5th grade. The absolute value of negative 5 equals positive 5. But that's not really what this is about. Instead, this term is trying to come with with the true, honest, real, and absolute value of a given asset. The term essentially tries to mash up the various valuation techniques of Wall Streety people...like Price-to-Earnings ratios, Enterprise-Value to EBITDA ratios, Discounted Cash Flow models, and on and on and on. And it's all a complete joke. There is no absolute in valuing stocks. Things are fluid. Much more like String Theory and astrology than a number line. So prayer is usually involved. And when you think about Absolute Value, well, when you're done, say, "Amen."

Acute Angles
angle less that 90°; not "the nice one"
Acute Triangle
a triangle with three acute angles (angles less than 90°) ; so adorable and petite.
A triangle that's slightly less cute than a-super-cute triangle. For that to be true, all the angles in the triangle have to be less than 90°.
A triangle with all three interior angles less than 90°. It may or may not be attractive.
A triangle whose angles are all less than 90 degrees. They have to shop in the petites section.
Adjacent
next to each other; you want to sit adjacent to the hot guy/gal
The conjoined twins of the geometry world. Adjacent angles always share a vertex and a side.
Adjacent Angles
angles that share a side
Two angles that share both a side and a vertex. They stick by each other through and through.
Alternate Exterior Angles
angles on opposite sides of the transversal and on the exterior of the parallel lines
The pair of angles on the outside of the two lines cut by the transversal and on alternate sides of the transversal. Alternate exterior angles are congruent if and only if the two lines crossed by the transversal are parallel.
Two angles that are on the outside of the parallel lines and opposite sides of the transversal. These types of angles are always congruent to one another.
The pair of angles on the outside of the two lines cut by the transversal and on alternate sides of the transversal. Alternate exterior angles are congruent if and only if the two lines crossed by the transversal are parallel.
The pair of angles on the outside of the two lines cut by the transversal and on alternate sides of the transversal. Alternate exterior angles are congruent if and only if the two lines crossed by the transversal are parallel. Also: as the name suggests, they're kind of hipster.
Alternate Interior Angles
angles on the opposite sides of the transversal and on the interior of the parallel lines
The pair of angles in between the two lines cut by the transversal and on alternate sides of the transversal. Alternate interior angles are congruent if and only if the two lines crossed by the transversal are parallel.
Two angles that are on the inside of the parallel lines and opposite sides of the transversal. These types of angles are always congruent to one another.
The pair of angles in between the two lines cut by the transversal and on alternate sides of the transversal. Alternate interior angles are congruent if and only if the two lines crossed by the transversal are parallel.
The stylish pair of angles that are in between the two lines cut by the transversal and on alternate sides of the transversal. They're congruent if and only if the two lines crossed by the transversal are parallel.
Angle
the shape formed when two rays meet at a common point (aka "the corner")
The corner of empty space between two lines, rays, or segments that share a point. Usually, angles are measured in degrees (and most of them have at least a bachelor's degree).
The space in between two intersecting lines. We measure the "wideness" of this space in units called degrees.
The separation in degrees of two lines with a common vertex. Any time lines cross, an angle is made. And when an angle is made, a math problem is born.
The corner of empty space between two lines, rays, or segments that share a point. Usually, angles are measured in degrees (and most of them have at least a bachelor's degree).
Angle measures the rotational displacement of an object from its original location. It depends on a defined axis the object rotates about, but otherwise behaves just like any angle you've encountered in a math class or previous physics chapter—180° is a half turn, 360° is a full turn. 1080° means you're probably Shaun White.
Apothem
The distance from the center of a regular polygon to the midpoint of one side.
The length from the center of a regular polygon to the center of one of its sides. If you look close, it is equivalent to the height of a triangle if you were to slice the polygon into the three-sided shapes.
Area
The amount of space inside the boundary of a closed shape. As in, "there is
x room to fit all the aliens inside Area 51."
The amount of space within the boundaries of a two-dimensional shape, reported in square units (like miles
2 or feet
2). Area is essentially space, but don't go around saying things like "area-ships," "area-cadets," or the "area-bar" on your keyboard.
The two-dimensional space contained by a particular region.
The amount of two-dimensional space that is taken up within a shape's perimeter.
A measurement of surface. It's like the amount of ground a piece of sod will cover, or exactly how much carpet we have to clean after spilling an entire crockpot of chili (oops).
The measure of the amount of space inside a polygon.
The amount of "stuff" inside of a figure. Very technical, we know.
Arithmetic Patterns
numbers in a pattern that are separated by a common difference
Base Of A Polygon
the bottom side of a polygon
Base Of A Solid
the bottom surface of an object; its tush
Basic Counting Principle
to find the total number of possible combinations multiply the number of options
Biased Questions
questions that try to influence the interviewee
Binomial
A polynomial with two terms. These guys always use the buddy system.
A polynomial with two terms.
A polynomial with only two terms. The expression
x – 3 is a binomial, and so is 96
x2y + 13,0278,543.
A polynomial with two terms. You can probably guess what trinomial means.
A polynomial with 2 terms. An example would be 5
x2 – 3.
Box And Whisker Plot
a plot that displays data broken into four quartiles, where the box represents Q1 to Q3 and the whiskers represent the extreme values
A representation of data that displays the range and quartiles of the data set. Looks like a kitty cat when you squint and tilt your head to the left.
A plot that depicts the four quartiles of data. No cat necessary.
A plot that clearly shows all of the quartiles in a set of quantitative data.
Cartesian Coordinate System
A system that has perpendicular axes, usually the
x- and
y-axis.
The flat grid we use to plot out functions. It has an
x-axis, a
y-axis, and a very big name.
A grid made up of two perpendicular number lines.
Chord
a line segment connecting two points on a circle; not "do re mi"
A line segment whose endpoints are both on a circle. Not a collection of musical notes.
Any segment from one point on a circle to another. Despite what you might think, they aren't all that musical.
Circle (Geometry)
A closed figure wherein points on the boundary are equidistant from the fixed center. More importantly, it's the shape of a pizza pie.
The set of all points in a plane that are exactly
r units away from point
O, where
r is the radius and
O is the center. The basis for such artifacts as wheels, wedding rings, and many types of cookies. We write "⊙
O" to denote "the circle with center
O."
A perfectly round two-dimensional shape. More technically, it's the set of all points that are the same distance away from another point (called the center).
A round conic defined by an eccentricity of 0. Also, a favorite shape for the terminally lost.
Common Denominator
the bottom part of fractions; in this case when more than one fraction has the same bottom as all the others
Commutative
when the order of the number doesn't matter; this works for addition and multiplication, but not subtraction nor division. 6 + 2 = 2 + 6 and 6 × 2 = 2 × 6
Complementary Angles
Angles that add up to 90°
Two wrongs don't make a right, but two complementary angles do. They're two angles that add up to 90° exactly.
Two angles that add up to 90°. Two wrongs don't make a right, but two complementary angles sure do.
Complementary Events
in probability, results that do not overlap with one another (when flipping a coin, if you get a tail, then the complementary event is getting a head)
A pair of mutually exclusive events where the occurrence of one implies the non-occurrence of the other; this is represented by the fact that the respective Venn diagrams for these events do not overlap, and that the two events themselves comprise the entire sample space.
Compound Events
in probability, when there is more than one outcome, which may (taking a second card after a first has been chosen) or may not (throwing two dice at the same time) affect the outcome of the other
These are when more than one event occurs. For instance, instead of picking one card from a deck, you pick two cards and find the likelihood of a King of Hearts and Queen of Diamonds being selected. Things are getting a bit more complicated with this one.
When more than one event happens at once. The lions, tigers, and bears all arrive at the same time? We think you know how that one ends.
Compound Interest
adding interest earned before calculating the new interest
A type of interest in which the money you acquire through interest
also acquires interest, which increases your money exponentially. Those two words should be music to your ears.
Com
pounding.
Like you start with a number—call it 4. You're growing at 10%. Then you com-
pound on it and it becomes 4.4. So now you forget 4. And you have 4.4. Then you compound again at 10% and it becomes 4.84.
That's geometric compounding. You pound on the latest number.
Conversely,
arithmetic compounding of interest (or any rate, for that matter), would just take 3 iterations of 4 and
add the 10%, meaning that you start with 4, then add 10% to get 4.4 and then add another 10% of 4 or 0.4 and you get 4.80.
Notice that in arithmetic compounding you ended up after only 3 compoundings .04 less. Too bad for you. Next time, go geometric if you wanna get big.
Cone
chocolate or brownie fudge? ; a solid with circular base and a curved side that ends in one point and has one vertex; a duncecap
A three-dimensional solid with a circular base and one vertex. We prefer to think of it as the waffle thing that ice cream comes in.
A 3D solid with a circular base and a curved surface that meets at a point. Essentially, a pyramid with a circular base.
An object that tapers from a circular base to a point. Just like a birthday hat (and sorry again about forgetting your birthday).
Congruent Angles
two or more angles that have the same measurement
Constant
A value that doesn't change, like pride in one's football team. Exception: the entire Philadelphia Eagles fan base.
A value that doesn't change. In a polynomial, the constant is the number that's not being multiplied by a variable, like the 4 in
x2 + 11
x + 4.
A value that does not change. Stay gold, Ponyconstant!
A number that doesn't change. Sometimes, we use this to mean a constant term, which is a number that isn't multiplied by any variables. In the expression 3
y + 6, the 6 is a constant term, but the 3 can also be thought of as a constant.
A number that doesn't change. Disproves the whole, "The only constant is change," idea, doesn't it?
A number that doesn't change in value. Like ½ or -7 or 38,501.
A value that does not change because it's old-fashioned and thinks everything is fine as is. When we look at an expression like 6
x + 2, the 2 is the constant.
A number whose value doesn't change. We call any freestanding numbers in an expression constants, because their values are fixed (in contrast to those shifty variables). In the expression 3
x + 7, 7 is a constant.
A number that is in a term all by itself. In the expression
y = 2
x – 16, 16 is a constant.
A term that is just a number, not multiplied by a variable or anything else. It's just along for the ride.
A value that never changes like pi or how much we love pizza. Mmm, pizza.
A constant is similar to a variable in that is exists in memory, but it can't be changed while a program's running. In C, a constant is declared using the keyword
const like this:
const float TAX_RATE = 0.10;
A name given to a value that never changes throughout a program. A constant is similar to a variable, except the constant's value never changes.
A quantity that doesn't change, usually of the number variety. These strong, steady quantities of the programming world can be trusted to send the same value every time, like pi, e, and our love of Cheez Whiz.
Coplanar
on the same plane
Used to describe lines or points that are all on the same plane.
Used to describe lines or points that are all on the same plane.
Correlation
how two variables relate to each other
The measure of the linear relationship between two variables. Can be positive or negative, depending on which side of the bed it woke up on.
If this is present, then there is an apparent trend in bivariate data. Correlation is generally positive or negative.
A relationship between two variables. They can be low or high, negative or positive, but if two things are correlated, they are more than just casual acquaintances.
When two variables we've measured have some kind of association or relationship between them. It can be positive, negative, or not exist. The last one is the most depressing, naturally.
When two or more events are related. This doesn't mean one caused the other, just that they happened at the same time, like wearing our lucky socks and acing the test.
A correlation is a link of some kind between two variables.
Most often the correlation is measured as part of a linear relationship between the two variables. Mathematically, correlation is the measure of how close the points on a scatterplot are to the line of best fit calculated using linear regression. The closer the points are to the line, the higher the correlation.
Correlations can be either positive (where, in general, both variables increase or decrease at the same time) or negative (where, in general, as one variable increases, the other decreases). A correlation of any kind does not, however, imply that the changes in one variable cause changes in the other to happen. We just know the variables that are correlated are linked somehow. We just don’t know exactly...how.
For example, ice cream sales and drowning deaths are highly, positively correlated. It’s pretty clear ice cream vendors aren’t murdering their clients once they get in the water, and in fact, both of these variables are very summer-dependent. In other words, in the summer more people buy ice cream, and more people are near water, cooling off and, sadly, drowning. Still, there is a definite correlation between increased ice cream sales and increased deaths by drowning.
When two or more events are related. This doesn't mean one caused the other, just that they are connected in some way—like wearing the lucky socks we haven't washed in a year and acing the test. In this case, the connection is that they happened around the same time.
Corresponding Angles
when a transversal intersects two lines, these angles are in the same position on each line. When a transversal crosses two parallel lines, corresponding angles are congruent
Two angles that are in the same relative place compared to each of the two lines and the transversal that cuts them. Corresponding angles are congruent if and only if the two lines crossed by the transversal are parallel.
A pair of angles that are in the same place relative to the transversal and their respective parallel line. They're congruent, too.
Two angles that are in the same relative place compared to each of the two lines and the transversal that cuts them. Corresponding angles are congruent if and only if the two lines crossed by the transversal are parallel.
These things are like two angle soul mates. They occupy the same relative position on their lines, compared to the transversal that cuts them. Corresponding angles are congruent if and only if the two lines crossed by the transversal are parallel.
Coterminal Angles
angles that share a terminal side
Angles that occupy the same position on the unit circle.
Angles that start and end at the same spots (usually start at θ = 0). They are different in the direction they travel or how many times they go around. (e.g. 270° and -90°, 30° and 360°).
Cross-Canceling
reducing the numerator of one fraction with the denominator of another when multiplying fractions; wearing bright orange on top might cancel the orange pants your date has on
Cube
a prism with six congruent faces, all right angles and parallel opposite faces; it is a form of a rectangular prism
A polyhedron made from six equal square faces. Also, the standard unit for ice.
Cylinder
a solid with two parallel circular bases; if you "unwrap" the middle section and lay it flat, it is a rectangle
Two parallel congruent circles whose circumferences are connected by a curvy rectangle.
A 3D solid that has two parallel circles for bases and a curved surface that connects them. Essentially, a prism with circles for bases.
A figure with parallel sides and circular bases. Picture a toilet paper tube. And for Pete's sake, if you finish the roll, replace it.
Denominator
A fraction's bottom. The fraction will usually try to keep this part of him covered up, but his mother will usually produce some scandalous baby picture of him in the tub where his denominator is clearly visible.
The bottom number of a fraction.
A fraction's bottom. The fraction will usually try to keep this part of him covered up, but his mother will usually produce some scandalous baby picture of him in the tub where his denominator is clearly visible.
The polynomial in the bottom of the rational expression. Be sure to keep it nonzero. Bad things might happen if not. Not that we're superstitious or anything.
The bottom half, or quotient, of a fraction. It's the ground level, supporting the rest of the fraction house on its shoulders.
In a fraction, the number on the bottom.
The bottom number in a fraction. It represents how many pieces the whole has been divided into.
The bottom number, or divisor, of a fraction.
Diagonal
a line connecting two vertices of a polygon
A segment that connects the two pairs of opposite vertices in a quadrilateral. In polygons with more sides, a diagonal connects any two vertices that are not right next to each other.
Diameter
the distance across the center of a circle
A chord of a circle that contains the center of that circle. Or, you know, the length of such a chord.
A segment from one end of the circle to the other. It's a chord that contains the center of the circle, or the length of that chord (which also happens to be twice the radius).
The distance across a circle, going through its center point. Also two times the radius. If you walk from one side of a crop circle to the other, you've walked the diameter. (But you'll probably be sucked into a UFO before you get there.)
Disperse
Spread or distribute over a wide area
to spread around
Distribute
to spread the term in front of the parentheses to each term inside the parentheses; share the wealth
Dividend
A number that is to be divided by another number. Apparently, this other number got hold of a butcher's knife.
The distribution of cash profits a company voluntarily makes to common shareholders at regular periods, usually quarterly in the U.S.
In division, a number that is being divided by another number called the divisor.
The amount to be divided up in a division problem.
Divisor
the number doing the dividing
The number which a dividend is divided by. Or, the number doing the dividing. Whichever way makes the most sense to you. Either way, there's going to be some good old-fashioned dividing going on.
In division, a number that divides another number called the dividend.
a number that another number will divide.
Edge
the intersection of two faces on a solid object; this is a line; "Livin' on the Edge"
A line segment that represents the intersection of two faces on a 3D figure.
Equation
A string of mathematical symbols and/or variables that states the equality of two algebraic expressions. Equations: bringing expressions together since 1931.
An expression that states the equivalence of two algebraic expressions.
Two expressions that have the same value, separated by an equal sign. They can play tug-of-war as much as they like, but the fact is they'll always be equal.
A mathematical statement that says two expressions are equal. Usually, the two expressions are separated by an equal sign.
A mathematical statement in which two different quantities have the same value. If it's got an equal sign (=) in it, it's an equation.
A complete math sentence. It connects two expressions using an equal sign.
A statement that two expressions separate by an equal sign are equal to one another. Get comfy with them, because pretty much all the math from here on out will be centered on them.
An equals sign with equivalent expressions on either side of it. Equations are the mathematical equivalents of a complete sentence.
When two expressions are just made for each other, they're exactly alike, they are equal.
A mathematical statement that says two expressions or values are equal to one another. You can tell a mathematical statement is an equation by the telltale equal sign.
A statement that says two expressions are equal to each other.
A mathematical statement that says two expressions are equal, used to represent a function or other relation.
Equiangular
a figure where all angles are equal in measure
Equilateral Triangle
a triangle with three congruent sides
A triangle that has three congruent sides. It should also be known that an equilateral triangle has three 60° congruent angles as well.
A triangle with all three side lengths that are equal. All three angles are also equal (all are 60°). If it has three of anything else, they're equal too.
Equivalent
equal to
Experimental Probability
probability calculated by the outcome of an experiment or trial
This is when we actually flip a coin, pull colored socks from the drawer, and so on, and record the results. This is real stuff.
A probability calculated from data over many trials of an experiment, and which represents the probability a given event in the sample space has of occurring.
The ratio of the number of favorable outcomes of a probability experiment to the number of trials. If we flip a coin twice and it lands on heads one of those times, that's a 0.5 experimental probability of landing on heads. If it landed on heads both times, that's a probability of 1.0.
Exponents
the power to which a number or expression is raised
Expression
a fragment of a mathematical sentence; it doesn't have a sign of equality
A collection of numbers, variables, and operations with no equal sign. Not the look on your face.
A mathematical quantity expressed in terms of constants and variables. No equal signs on these bad boys, since they represent a single value.
A mathematical phrase containing numbers and operators, but no equal sign. For you English buffs out there, they're a clause, but not a sentence.
A series of terms representing a quantity. Speaks only in emoticons.
Extreme Values
the largest and smallest values in data set; think extremes - extreme sports are at the high end of danger
Values that are more than 3(IQR) away from the outer quartiles; shown as open circles on a box and whisker plot.
Face
a flat side of a 3-dimensional object
The flat side of a 3D figure.
One of the surface shapes that forms a 3D solid, or what your Dad shaves every morning…or most mornings.
Factor
A number that divides evenly into another number. For example, 8 and 3 are factors of 24. Oh great—now we're really gonna hear it from 2, 4, 6 and 12. We said
for example. Sheesh.
A number that divides evenly into another number. For example, 8 and 3 are factors of 24. Oh great—now we're really gonna hear it from 2, 4, 6 and 12. We said for example. Sheesh.
Any number or variable that is multiplied by something else. Since 1 counts as a factor even when it's not written out, every term (see below) in an expression has at least two factors.
A factor is any integer that you can multiply by another number to get a specific product
n. If a set of numbers (two or more) has a product equal to
n, we say they are all factors of
n.A thing that's multiplied by another thing. For instance, 3
x has two factors: 3 and
x. They're partners in crime, and the crime is making a larger number.
An algebraic term or constant that is multiplied by another to make a third term. Kind of like how a snowman is made up of three factor snowballs.
Factors are the inputs firms need to make the stuff they’re selling, which includes labor.
Just like consumers have the consumer market, firms have their factor market. The main factors of production that firms need to make things are land, labor, capital, and raw materials.
Demand for flour in the factor market is, in part, derived from the demand for croissants in the consumer market.
The firm demand for battery engineers? It’s derived from consumer demand for longer-lasting batteries. Because having your phone die at the worst time is, well...the worst.
Demand for rubber on the rise by firms during the Baby Boom era? Maybe because the increase in supply of babies led to an increase in the demand for rubber duckies, which increased factor demand for rubber.
Or maybe firms were making something else with the rubber…hmmm…

)
The explanatory variable in our experiment. We create treatments made of our factors. Sometimes, they come in different levels. Sometimes, they don't.
Factorial
the product of all positive integers less than or equal to the given number; 5! = 5 × 4 × 3 × 2 × 1 = 120; a very excited number
The product of all the integers from
n down to 1:
n! =
n × (
n – 1) × (
n – 2) . . . × 2 × 1. The most excited of all key terms.
The product of a number and all the numbers before it, all the way down to 1. For example, 5! would be 5 × 4 × 3 × 2 × 1.
When you see the !, multiply the original number by one less than that number times one less than that number, until you get all the way to the number 1. For instance, 5! = 5 × 4 × 3 × 2 × 1.
A number multiplied by every positive integer less than itself. n! = n × (n – 1) × (n – 2) × … × 2 × 1.
The product of an integer and all the positive integers preceding it, is represented by a ! symbol. For example, 4! (“4 factorial”) is 4×3×2×1. The definition of 0! is 1. Weird.
Fauna
Animals of a particular habitat or time period
Finite Decimals
decimals that have an ending; unlike that dreadful movie from last weekend that seemed to never end
Frequency
the number of times an event occurs; Jim asked Danielle out with uncommon frequency
Frequency is the number of wavelengths that pass a specific point in a certain time period.
Frequency is the number of complete oscillations per second made by a wave, measured in hertz (1 Hz = 1 s
-1). In the basic solution to simple oscillation,
x(
t) =
Acos(ω
t + φ), the variable ω represents a wave's
angular frequency, which is measured in rad/s. Angular frequency can be converted to frequency by dividing by 2π (the number of radians in one cycle):
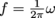
. Frequency—normal frequency, not angular frequency—is the inverse of the period of the wave:
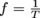
. The frequency of a wave is also inversely related to the wave's wavelength:

. Higher frequency light has more energy than lower frequency light, and looks more blue than red. Higher frequency sound is higher in pitch than lower frequency sound. Higher frequency flyer miles can get you a better seat on selected domestic flights.
The time it takes for a wave to pass through a specified point. The higher the frequency, the shorter the wavelength.
Frequency is the number of complete oscillations per second made by a wave, measured in hertz (1 Hz = 1 s
-1). Frequency is the inverse of the period of the wave:
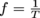
. Higher frequency light has more energy than lower frequency light, and looks more blue than red. Higher frequency sound is higher in pitch than lower frequency sound. Higher frequency flyer miles can get you a better seat on selected domestic flights.
Geometric Patterns
numbers in a pattern that are separated by a common ratio (number being multiplied)
Golden Ratio
a ideal proportion that occurs regularly in nature, architecture, and art; approximately 1.618 (not 6'0", 185...)
The ratio that is seen in nature and art. It makes structures look proportional.
Greatest Common Factor (GCF)
The largest positive integer that divides evenly into two or more non-zero numbers. For example, the GCF of 18 and 24 is 6.
It's also known as the greatest common divisor or highest common factor. It goes by many names, and has a different passport for each. How Jason Bourne is that?
The largest positive integer that evenly divides (with no remainder) two or more nonzero numbers. It loves nicknames, so it's also known as the
greatest common divisor or
highest common factor. For example, the
GCF of 18 and 24 is 6.
The GCF is the largest factor that two numbers share.
Hexagon
a six-sided figure
Hexagonal Prism
a prism with hexagons for bases; opposite faces are parallel
Hypotenuse
The longest side of a right triangle. It'll always be opposite the right angle.
Looks a little like the word hippo, so remember hippos are big and the "hypo" is the biggest side of the right triangle. It's the side opposite the right angle.
The longest side of a right triangle, or the one directly across from the right angle. It's the star of the show, and the triangle legs are its backup singers.
Improper Fraction
A fraction that tells bawdy jokes in mixed company.
Oh, all right. You're no fun. An improper fraction is one in which the numerator is larger than the denominator, like 13/5 or 25/4. These can be expressed as mixed numbers. Keeping with the examples, these fractions could be written as 2 3/5 and 6 1/4, respectively. There, now our fractions aren't so top-heavy.
A fraction whose denominator is smaller than its numerator. It's a little top-heavy. An example is
4/
3.
A fraction where the numerator is greater than the denominator. They usually have really poor table manners, too.
Inequality
A relation between two algebraic expressions using the symbols <, >, ≤, and ≥.
A relation between two unequal algebraic expressions using the symbols <, >, ≤, and ≥.
A relation between two algebraic expressions that aren't equal. It uses the symbols >, <, ≤, and ≥.
A mathematical statement using the symbols <, >, ≤, ≥.
A mathematical statement using the symbols <, >, ≤, ≥.
A mathematical statement that says two expressions aren't equal in some way. Sometimes, it's a little more specific (like saying one expression is greater than or less than the other).
A mathematical statement in which two different quantities do not have the same value. We can use symbols like greater than (>), less than (<), or not equal to (≠) for inequalities.
A mathematical statement using the symbols <, >, ≤, ≥. Like not equal, man.
A relation between two algebraic expressions that are not equal, expressed using symbols like <, >, ≤, ≥. However, those expressions are currently marching on Washington, and hopefully, someday soon, there will be equality for all.
Similar to an equation, these have > or ≥ or < or ≤ instead of an equal sign.
A mathematical expression that uses ≤, ≥, <, or ˃ to define a relationship between two groups. It's not the opposite of an equality. It's like a more inclusive one.
An expression showing the way in which two things are not equal. Symbols are used to demonstrate the relationship between the quantities. Sorry if they cause flashbacks to the time your sister got more ice cream than you did.
A mathematical statement comparing two quantities
The inequality signs < and > tell us that one number is less than or greater than another, respectively. They could be hungry mouths, or accusing arrows. Or maybe something even scarier.
A statement that compares two expressions and says that one expression is greater than the other, using less than (<), greater than (>), less than or equal to (≤), or greater than or equal to (≥) signs.
A mathematical expression that uses ≤, ≥, <, or ˃ to define a relationship between two groups. It's not the opposite of an equality. It's like an equality with extras.
Integers
natural numbers (1, 2, 3, 4,...), their negatives (..-4, -3, -2, -1) , and zero; from the root "untouched"; simply virginal numbers
Integers are positive or negative whole numbers, including 0. They have a big "No Fractions Allowed" posted on their clubhouse.
The inequality signs < and > tell us that one number is less than or greater than another, respectively. They could be hungry mouths, or accusing arrows. Or maybe something even scarier.
Intercept
the place where a line or curve crosses an axis
The point where the graph of a function crosses the
x-axis or
y-axis.
The point where the graph of a function crosses the
x-axis or
y-axis.
For all you sports nuts—think football. It's the point where a graph "takes," "seizes," or "crosses" an axis.
This is the place where a graphed equation hits the
x- or
y-axis. It's also the point at which
x = 0 or
y = 0.
The point where the graph of a function crosses the
x-axis or
y-axis. Slow down line. The axis gets the right-of-way.
A point on a graph where a line or plane crosses one of the axes.
The point where the line crosses the
x- or
y-axis. Hope it looks both ways first.
The place on an axis where the graph intersects it. A spot where the graph crosses the
x-axis is called an
x-intercept; anywhere it crosses the
y-axis is a
y-intercept.
Interquartile Range
the difference between the upper quartile and the lower quartile
The difference between the third and the first quartile.
The difference between the first quartile and the third quartile.
The middle 50% of a data set, located between Q1 and Q3. It's a club with a 50/50 acceptance rate, you could say.
The range of the middle 50% of the data. Interquartile range is represented by IQR = Q
3 – Q
1.
Isosceles Trapezoid
a trapezoid with only one set of congruent sides and two sets of congruent angles and has all the characteristics of a trapezoid (see trapezoid)
A trapezoid whose two non-parallel sides (legs) are congruent. Its two pairs of base angles are also congruent, much like those of an isosceles triangle.
Kite
a quadrilateral with two sets of adjacent congruent sides and only one set of congruent angles
A quadrilateral with two distinct sets of congruent consecutive sides. (They share an angle.) It has perpendicular diagonals and is perfect for windy days.
A quadrilateral with two pairs of congruent consecutive sides and only one pair of congruent opposite angles. You'll recognize these guys because…well, they look like kites.
A quadrilateral with two adjacent pairs of congruent sides. It's also got perpendicular diagonals and an area formula of
A = ½
d1d2, where
d1 and
d2 are the diagonals. Colorful tail and windy days not included.
Least Common Multiple (LCM)
The smallest integer that is a multiple of two or more integers. For example, the
LCM of 4 and 6 is 12. If you take 36, on the other hand, it's a multiple of both numbers, but it is not the
least common multiple. Don't get down on yourself, 36. You'll have your day in the sun.
The LCM is the least common multiple that two numbers share.
Like Terms
Two terms that have the same variables raised to the same exponent. Like terms may have different coefficients. We give them permission.
Two terms that have the same variables raised to the same exponent. Like terms may have different coefficients.
Two terms that have the same variables raised to the same exponent. Like terms may have different coefficients.
Two terms with the same variables raised to the same exponent. Like terms may have different coefficients.
Terms that have the same variables raised to the same exponents.
Terms that are made up of the same variables and the same exponents. They play nice together because they "like" each other.
Terms that can be added or subtracted together. They'll always have the exact same variables with the exact same exponents, like 8
x2y and -3
x2y. They go together like crunchy peanut butter and smooth peanut butter.
Any terms that have the exact same variable, raised to the exact same power. Accept no substitutions. Thanks to their identical variables, like terms can be added to and subtracted from one another: 2
x2 + 5
x2 = 7
x2.
We do like "terms," but that's not what this term means. "Like terms" refers to all the terms that contain the same variables and so can be added together.
Terms in a polynomial that exactly match their variables and the exponents for each variable. The coefficients may be different.
Two terms with the exact same radical. If the stuff inside the radical (the radicand) matches, we can combine those terms just like we do with like variables.
Line
a straight path passing through at least two points that extends in both directions; imagine the fifty yard line going on forever
A line is a unit of poetry that takes up—you guessed it—a line of text. It's not a unit of sense or meaning (although it can be if the lines are
end-stopped). It's a unit of form.
And now, Shmoop will regale you with recitations of our top ten favorite lines of poetry. Ever.
She walks in beauty like the nightL'amor che move il sole e l'altre stelleThere is no Frigate like a BookJazz June. WeI am large . . . . I contain multitudes.The blackbird must be flying.Te amo sin saber cómo, ni cuándo, ni de dónde,Lose something every day. Accept the flusterWhat form my dreaming was about to take.Time's wingèd chariot hurrying near;
Okay, okay, eleven:
I have measured out my life with coffee spoons;A one-dimensional segment that continues on forever in both directions. Time-consuming to draw, so we use arrows on the ends to symbolize that it never ends.
A unit of poetry that goes in a straight...line.
A unit of poetry that goes in a straight...line.
See also line.
An infinite length. We usually draw them as straight lines with arrows on either end to indicate that it goes on forever in both directions. Lines are one-dimensional since they're only length without depth or width.
A perfectly straight connection between any two points, extending out to infinity in both directions, with no thickness. It sounds weird spelled out like that, but it's just the mark you make with a ruler.
A one-dimensional bar that continues on forever in both directions. Time-consuming to draw, so we use arrows on the ends to symbolize that it never ends.
A unit of poetry that goes in a straight… line.
Line Segment
a portion of a line that has limits at each end; think football field - there is an out-of-bounds at each yard line
Sometimes just called a "segment." It's a finite piece of line between two endpoints.
A measurable piece of a line. Rather than continue on forever, line segments are one-dimensional lengths caught between two endpoints.
A measurable piece of a line. Rather than continue on forever, line segments are one-dimensional lengths caught between two endpoints.
Linear
in a straight line
An equation or graph whose rate of change is constant over time. They're on the straight and narrow path and they have no plans to switch things up anytime soon.
A function that changes at a constant rate. They found something that works for them and they see no reason to change things up.
Something to do with equations, maybe? Yes, if you're talking math, but not so much in literature. A linear plot is one that goes from one event to the next in the order they happen—no flashbacks or flashforwards, and probably not a lot of subplots. It's linear because if you diagrammed the plot, it would look like a straight line. (Sadly, there are no
geometric plots—except maybe in
Flatland.)
Mean
the sum of all numbers in a set divided by the number of data values
Also known as the average; found by adding all of the numbers up, then dividing by how many there are.
The average entered the witness protection program, and this is its new name. Although now that we've told someone, that kind of defeats the purpose of the whole thing. Whoops.
The average of all your data points, or the sum of their values divided by the number of values.
The average value of the data, or all the points added up, then divided by the number of points there are. Contrary to the name, it's very agreeable.
The opposite of nice.
In finance, it's basically a fancy word for "average."
The mean is the average score of distribution; obtained by adding scores together and dividing by number of scores.
The average of a dataset; this is
not, however, what your sister calls you after you use all of the hot water for your shower.
The average of a set of quantitative data. To find it, add up all of the data points, then divide by the total number of points we added together. It's quite a bit nicer than its name would indicate.
The average of a set of data. We find this by adding up all of our numbers, then dividing the sum by the number of data points we have. This has nothing to do with our lab partner's attitude.
The average value of a collection of numbers.
The average of a set of numbers. We calculate it by adding the numbers up and dividing their sum by how many numbers we have. It gives us a good idea of what's in the middle of a set of data, or what's "normal."
The average of the data. It's quite a bit nicer than its name would indicate. To find it, just add up all of the data points, then divide by the total number of points we just added together.
Median
the middle value in a data set
The middle value of a list of data points.
A segment parallel to the bases of a trapezoid that connects the midpoints of the non-parallel sides. A trapezoid's median also has a length that's the average of the two bases.
The middle value in a data set when all of the values are lined up in order.
The line from a vertex of a triangle to the midpoint of the opposite side.
The middle value of a dataset. Like, even moreso than the mean.
The value of the middlemost data point. If you arrange all your data points in increasing order, it'll be the one smack-dab in the middle.
The center value in the data, or one with an equal number of points smaller than and greater than it. It's the bullseye on the graph dartboard.
Here's a series of numbers: 2, 3, 5, 5, 5, 7, 7, 8.
The median is 5.
Why?
Click here.
The median is middle score in a number of scores.
The middle term or number of a dataset,
not the thing you've run over several times in the middle of the highway.
The middle of a set of quantitative data listed in order from least to greatest. Actually, it doesn't matter whether we go least to greatest or greatest to least. The middle is all cool and stays in the same place either way. If there are an even number of values, the median is the average of the middle two values.
The middle number in a dataset. Sometimes there are two, so we take the mean of them to get the median.
The middle value of a collection of numbers.
If we line up our data from smallest to largest, the median is the number smack dab in the middle. If there is no middle (like when we have an even number of data points), we just take the mean of the two numbers in the middle and, voila, a median is born.
Mixed Number
A number expressed as a whole number
and a fraction, like 2½ or 4¾. You'd never be able to bake a cake without these bad boys.
No only is there a fraction, but there's a whole number attached, too. An example is 4
1/
3.
A number that's written as the combination of a whole number and a proper fraction.
Mode
the number that occurs the most in a data set
The data point with highest frequency.
The value that shows up the most in a set of data.
The most common value in a dataset. The mode of a beach is "sand," the mode of Alaska is "snow," and the mode of Shmoop is "fun."
The most common response. If your data set were a graduating class, the mode would be the student voted Most Popular.
This video says it all. Check it out:
The item that appears the most in a dataset,
not whether your TV is set to watch cable or use Netflix.
The data point or points that occur most often. This is also what we like our pie à la.
The number that shows up the most frequently in a dataset. This repeater is a good indicator that the data are precise.
The number that shows up most often in a collection of numbers.
This is the number that shows up the most in a set of data. Yes, it's possible to have no mode or even more than one mode. It tells us if certain numbers are more common than others so we can look for patterns in a set of data.
Monomial
an algebraic expression with one term
A polynomial with one term.
A polynomial with one term. Single and ready to mingle.
An algebraic expression with only one term. That term could be
x or 7 or 18,942
x13y8.
A polynomial with one term.
A polynomial that's just a single term, like 5
x or -16
xy2z. Aww, it's so widdle and cute.
Mutually Exclusive Events
in probability these are two or more outcomes that can't occur at the same time (like rolling a die and getting a 1 and a 3)
Events that cannot both occur at the same time.
Two or more events that can never occur at the same time; this is represented by the fact that their respective Venn diagrams do not overlap.
Two events A and B are mutually exclusive if they share no outcomes. Never the twain shall meet.
Events with no outcomes in common. They're events that can never happen together, like getting heads and tails on one coin flip, or rolling a 6 and an odd number on a single die roll. Or being first in line for Wisconsin Cheesy Chicken in the cafeteria and being anywhere else in line for the Wisconsin Cheesy Chicken.
Negative Correlation
as one variable increases, the other decreases; like hours spent on homework and the amount of time your parents nag you
See: Correlation. Multiply by negative one.
You know those correlations that always rain on your parade, or that always assume the worst is going to happen? That’s not this kind of negative correlation, but those guys are real downers, aren’t they?
A negative correlation is a linear-ish relationship between two sets of data characterized by the pattern that, as the values in one data set increase by a certain value, the values in the other data set decrease by a certain (and usually different) value. Like, there’s a negative correlation between how long we’re on Reddit and the number of chores we get done.
Something highly negatively correlated means that one action has the opposite reaction of the initial one. Pounds of chocolate eaten this month is negatively correlated to the number of packs on an otherwise 8-pack stomach.
A set of data that have a downward trend. Everybody grab your sleds.
No Correlation
the variables have no relation; like hours spent on homework and height
When the scatterplot doesn't have a wholly upward or downward trend or has both trends or is just a huge glob o' dots smeared all over the plot.
Nonagon
a nine-sided figure
Numerator
The top part of a fraction.
"I'll be back... on top of the denominator." -
Numerator II: Judgment DayThe top part of a fraction. "I'll be back... on top of the denominator." - Numerator II: Judgment Day
This is the polynomial in the top of the denominator.
The top number in a fraction, or the dividend. It's the attic space holding the group of things we need to divide. And a lot of spiders.
In a fraction, the number on the top.
The top number in a fraction. It represents the number of parts included in the fraction.
The top number, or dividend, of a fraction.
Obtuse Angle
an angle greater than 90°, but less than 180°; a not-very bright angle
An angle greater than 90°. Or just a really thickheaded angle.
An angle greater than 90°. Or just a really thickheaded angle.
Obtuse Triangle
a triangle with one obtuse angle (an angle greater than 90°)
A triangle that's a little slow on the uptake. Or one with an angle that's over 90°.
A triangle with one angle greater than 90°. It may or may not be intelligent.
A triangle in which one of the angles is more than 90 degrees. It's like a normal triangle someone sat on and squished a little.
Octagon
an eight-sided figure
Order Of Operations
The rule that states which operation takes precedence over others. The correct order is given by the acronym "PEMDAS," which can be remembered by using the mnemonic "Please Excuse My Dear Aunt Sally." It stands for "Parentheses, Exponents, Multiplication and Division, Addition and Subtraction." It's something like a ranking system or a chain of command. So an exponent had better never go over a parenthesis' head, or it might be cited for insubordination.
All bow before mighty PEMDAS: Parentheses, Exponents, Multiplication, Division, Addition, and Subtraction. Execute your operations in this order, or else PEMDAS will have a stern word or a thousand for you.
Outliers
a number that is far greater or smaller than the rest of the data; it is calculated as 1.5(IQR) > Q3 or 1.5(IQR) < Q1; hopefully not your math score on the low end
Data points that are numerically far away from the rest of the data set. The loners of the group, if you will.
Data points that don't go along with the trend. They like to stand out from the crowd.
Values that are more than 1.5(IQR) away from the outer quartiles (the edges of the box on a box and whisker plot); shown as asterisks on a box and whisker plot.
A data point that is very far away from most of the rest of the data. It gets a bit lonely sometimes.
Parallel Lines
lines that lie on the same plane and never intersect (Labeled as JK || LM )
Two lines that never ever intersect. They can continue on forever, but they'll always stay the same exact distance apart.
Lines that will never intersect, because they share the same slope.
Two lines that are on the same plane but never intersect. They're always in sight, but never touch…sort of sad, ain't it?
Parallelogram
A four-sided, closed shape with straight lines and two pairs of opposite sides that are parallel. You can send someone a parallelogram for his or her birthday, but it's not as entertaining as a sing-o-gram.
A quadrilateral in which both pairs of opposite sides are parallel. Consequently, they're also congruent. And their opposite angles are congruent. And their consecutive angles are supplementary. And their diagonals bisect each other. And they like long romantic walks on the beach and reading Danielle Steel novels.
A quadrilateral with two sets of parallel lines. It's not called a parallelogram for nothing, you know.
A quadrilateral with two sets of parallel sides. To track down a parallelogram's area, we bust out the formula
A =
bh, where
b is the base length and
h is the height. Careful though; the height might not necessarily be one of the sides. Tricky, tricky.
Percent
The portion of a number out of 100. We're sure you can grasp this concept if you just give it 110%.
A number that expresses a ratio out of 100. If you've ever been to a department store or taken a test, you've seen percents in their natural habitats. We're 100% sure of it.
A specified amount for every hundred. It's what happens when the denominator of every fraction is converted to 100. It's like they're all wearing matching pants.
Means "out of a hundred." The value of a percent can be expressed as a fraction with a numerator out of 100. Percents can also be converted to decimals by moving the decimal place two spaces to the left.
Percents represent part-to-whole relationships that have been converted into a ratio where the whole is equal to 100. They can be written as fractions with 100 in the denominator, as percents, or as decimals.
Perimeter
The length of the boundary of a closed shape. If the boundary is a light, bluish-purple and you can only see it out of the corner of your eye, it's a peripheral periwinkle perimeter. Just in case that ever comes up.
The length of the boundary of a closed shape.
The total distance around a two-dimensional figure. We can calculate the perimeter for any figure by adding up all the side lengths together.
The distance around the edge of any shape (or the sum of its side lengths). "Patrolling the perimeter" just means walking around the edge. We've been doing it wrong this whole time.
The measure of the total distance around a polygon.
Perpendicular Lines
lines that intersect at a 90° angle; a linebacker's path while running at the quarterback
Two lines that intersect at a right angle. Actually, they make
four right angles.
Two lines that create a 90° angle when they intersect. Well, actually they create four 90° angles, but who's counting?
Pi
apple or cranberry? the ratio of the circumference of a circle to its diameter, 3.14159...; impress family and friends by memorizing to at least 10 digits
Place Value
each digit in a number has its own place; think Thanksgiving dinner; the ones place is to the left of Grandpa (or the decimal), tens place is far down from Aunt Gert
Plane
a flat surface without boundaries (Labeled by naming three nonlinear points on the plane,
A "slice" of three-dimensional space. It has length and width, but no depth, like a sheet of paper that stretches out forever in all directions.
A two-dimensional region that has length and width, but no depth. Think of a sheet of paper that's both infinitely thin and extends in all directions.
A "slice" of three-dimensional space. It has length and width, but no depth, like a sheet of paper that stretches out forever in all directions.
Point
a single location usually drawn as a dot; "dimensionless" (labeled as point P)
The smallest object…ever. It has no mass, no length, and no size. It describes only a location.
A single location in space. Even though we represent it using a dot, it technically has no dimensions and no size.
An exact position on a plane, like a zit on a chin. It has no size or dimensions (unlike that zit).
A single location in space. Even though we represent it using a dot, it technically has no dimensions and no size.
An (
x,
y) location in coordinate space. Just like in all your math classes, it’s really just a dot in a grid.
Polygon
a closed figure of three or more sides
A closed two-dimensional shape that's made of only straight line segments. No curves allowed. Sorry, Beyoncé.
A closed 2D shape that's made up entirely of straight line segments. No saucy curves on these guys. They're all lines and angles.
A closed 2-D shape that is made up entirely of segments. No saucy curves on these guys. They're all lines and angles.
A flat, closed 2D shape made of straight lines.
Positive Correlation
as one variable increases so does the other; like hours spent putting and accuracy (we hope)
See: Correlation. This is the, uh...upbeat variety.
When we have two variables that are plotted against each other on a scatterplot and we see a linear-ish pattern running from the lower-left to the upper-right, the two sets of data will have a positive correlation. This positive correlations says that, in general, as one variable increases by a certain amount, the other variable also increase by a certain (but not necessarily equal) amount.
It also holds true that, in general, when one variable decreases by that certain amount, the other variable also decreases by a certain (but not necessarily equal) amount.
Things that are positively correlated? The number of hours we spend watching Futurama...and our performance in online Futurama trivia contests.
The price per barrel of oil and the price per gallon of gasoline.
The time we spend thinking up jokes and the quality of said jokes.
A set of data with an upward trend. Time to grab the climbing gear.
Powers
math "shorthand" devices used to make writing long multiplication expressions easier and faster (also see exponents)
Prime
a number that is only divisible by one and itself; the first ten prime numbers are 2, 3, 5, 7, 11, 13, 17, 19, 23, and 31
Prism
a solid object with two congruent and parallel faces
Two parallel congruent polygons connected by lateral faces. Not "prison" in a French accent.
A 3D solid with two polygonal bases that are parallel and rectangular faces connecting them.
Probability
the likelihood of an event occurring
Likelihood or chance of the occurrence of an event.
This is the study of how likely an event is to happen.
How likely something is to occur;
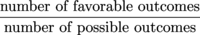
Product
A molecule that is produced in a chemical reaction. Products are generated by reactants.
The chemical compounds resulting from a chemical reaction. In a chemical equation, the products are listed on the right hand side of the arrow.
In a chemical reaction, the product is the chemical outcome. It appears to the right of the arrow in a chemical equation.
The compounds that exist after the conclusion of a reaction. It's also the new molecules that are formed as a result of a chemical reaction.
The result of multiplication. For example, the product of 3 and a number is 3
x. It's what gets made at the end.
The new compounds formed when a reaction takes place.
A molecule that is produced in a chemical reaction. Products are created by reactants. However, products can also be reactants, since biological reactions are reversible.
Proportion
a comparison between one part and the whole
A mathematical statement that establishes equality between two ratios. By calling themselves proportional, ratios proclaim to all that they are equivalent and deserve a nice crusty baguette just as much as the next ratio. "Liberté, égalité, geometré," as Victor Hugo so wisely wrote.
A single ratio comparing a part to a whole or two equivalent ratios. In the first case, the "part" is always the first number (the numerator in the fraction) and the "whole" is the second number (the denominator). When referring to equivalent ratios, it's most commonly written as two equal fractions.
This means there is some relationship between two things, as compared to the whole.
The relationship between two equivalent ratios. When doubling a recipe, it's important that the new ingredient amounts be in proportion to the original amounts.
A comparison to show a relationship between parts and each other or parts and a whole. The proportion of the cherry pie we ate compared with the whole pie was very large.
Proportional
having a constant ratio; similar figures are proportional
Corresponding in size or amount to something else. It's like how our model trains are all in scale with the real deal (but have many fewer delays and unruly passengers).
Two things are proportional if their ratio is constant. Those things can be parts of shapes, like side lengths, which makes the shapes similar.
A relationship where a number either increases at the same rate that another increases or decreases at the same rate as another decreases.
Pyramid
a solid object with a polygon for a base and triangles for sides
Those huge stone buildings that the Egyptians built. It's basically a solid (definitely not a liquid or a gas) with a non-curvy shape for the base and one tip at the top.
A 3D solid with a single polygonal base and several triangular faces that meet at a single vertex.
A figure with a polygon base and triangular sides that meet at an apex. Generally full of old organs in jars.
Q1
quartile 1; the median of the lower half of the data set
Q2
quartile 2; the median of the entire data set
Q3
quartile 3; the median of the upper half of the data set
Quadrilateral
four-sided shapes
A polygon with four sides. We can remember this easily because "quad" means "four" and "lateral" means "side."
A two-dimensional figure with four sides.
Quartile
one of three values that divide a data set into four equal sections
One of three values that divide a data set into four equal sections.
Not the coin. Not the periods of either football or basketball games. Instead a quartile is a divider in a data set. We take the numbers in a data set, numerically order them, and then divide the data set into four equally-sized groups of data points. The three points that are the dividers mark the quartiles.
They’re located at 25% of the data, 50% of the data, and 75% of the data. The quartile at 25% is called Q1, the 25th percentile and the first quartile, and it’s the top end of the data running from the lowest data point to Q1. The quartile at 50% is called Q2, the 50th percentile, and the median (yes, that median...the middle of a data set), and it’s the top end of the data running from Q1 to Q2. The quartile at 75% is called Q3, the 75th percentile, and the third quartile, and it’s the top end of the data running from Q2 to Q3 as well as the lower end of the data running from Q3 to the largest data point..
Numbers that divide a dataset into 4 equal quarters. We mostly use them to ride the mechanical horses outside the grocery store.
Quotient
The number obtained by dividing one number by another. Did you know that I.Q. stands for Intelligence Quotient? That's because we can tell how smart you are by dividing your brain up into pieces. Sounds rough, but it's a relatively painless procedure.
The divisor in a division problem, or the number of groups the problem creates. If we are dividing the episodes of a TV show into seasons, it's the number of seasons we have.
The result of dividing a dividend by a divisor. For example, 2 is the quotient of 8 divided by 4.
The answer of a division problem. It's what we get out when a fraction goes through a simplifying steamroller.
Radius
the distance from the center of a circle to a point on the circle
A line segment with one endpoint at the center of a circle and the other endpoint on the circle itself. Alternatively, the length of such a segment. (The plural is
radii, pronounced "raidy eye.")
A segment from the center of a circle to any point on that circle. Sometimes it just describes the length of that segment.
The length from the center of a circle to any point on its edge. It's the distance from the cheesy tip of a pizza slice to the crust. (And if you're not planning to eat that, slide it our way.)
Range
The difference between the highest and the lowest data value; on a golf driving range, the difference between your ball and that of Tiger's might be large
The set of all possible outputs (
y-values) of the function.
The geographical area an organism lives in. The range is the extent of land (or water) that a species occupies. Species periodically expand their ranges, just naturally or because of changes in habitat and climate. Before 1850, the Rio Grande was the northern range limit for armadillos. Since then, armadillos have moved further north into the US, but no one knows why. Many species ranges are expected to shift because of climate change.
The output values of a function or relation.
This is the set of all possible outputs of a function. It's generally the possible
y-values. It has very little to do with the Lone Ranger or free range chickens.
The difference between the highest value and the lowest value in a data set.
The set of
y values for which a function is defined. It's anything that comes out the other end when we put in one of our domain values.
The set of all possible outputs,
y, for a given function. The impossible outputs are in the don'trange. Wait, nevermind, that joke doesn't work.
The set of values that the dependent (
y) variable can have.
The possible outputs of a function. It's like all the possible meals you can make with what's in the pantry. (We've got tuna, peanut butter, and lentils, and we are taking suggestions.)
All the values
f(
x) can take, based on the domain. Just how high and low does it go?
Home, home on the... yeah.
The term also refers to the prices in which, say, an
IPO is expected to be priced. That is, you'll find on the cover of the
Red Herring IPO marketing document an "expected range of $12–$14," for example.
The term can also apply to, say, the 52-week trading range in which Stock X has traded between $15 and $24 a share.
The term also means the difference between the highest and lowest numbers in a group.
The term also refers to the thing you cook on...
Actually, the term means too many things. It should stop hogging all the definitions.
Related to, but not equivalent to, a firing range. This is the maximum horizontal distance achieved by a projectile.
How much variation there is in our data, or the area between the highest and lowest values. Not to be confused with where the deer and the antelope play.
The difference between the largest and smallest numbers in a data set. Yes, you'll have to do a little subtraction.
Ratio
a comparison between two or more quantities; the ratio the distance Drew Brees throws the football over the distance you throw it is probably a high number
A comparison of two quantities, written as a fraction (
 ), with the word "to" in between (3 to 5), or with a colon (9:2). Not to be confused with CSI: Miami's Horatio.
), with the word "to" in between (3 to 5), or with a colon (9:2). Not to be confused with CSI: Miami's Horatio.Two numbers compared to each other. We can represent ratios as fractions or two numbers with either a colon or the word "to" in between them. The order of the numbers is important!
The amount of times one value occurs in relation to another value.
Relative sizes of two or more values. Usually presented looking like a fraction. But don't let them fool you! Their bottoms don't represent the whole.
The relationship between the sizes of two groups. A ratio may compare groups of two different things (two mushrooms to every anchovy on a pizza), two groups of the same type of thing (for every kitten Jim has, Marv has two kittens), or the size of a subgroup compared to the size of the whole group (the number of green M&Ms in a bag relative to the total number of M&Ms in the bag).
Ray
a straight path with one terminal point and extending indefinitely in the other direction; think sunshine
A hybrid of a line and a segment. It has one endpoint, but then goes off forever in the other direction. It's like a ray of sunshine that starts at the sun and then continues on forever.
A segment that has one endpoint but extends forever in a single direction. Think "ray of sunshine," and not "stingray."
A line with one endpoint, shooting infinitely in one direction. It's like a ray of sunshine shooting off from its endpoint on the sun and giving you tan lines.
A segment that has one endpoint but extends forever in a single direction. Think "ray of sunshine," and not "stingray."
Rectangle
A parallelogram with all angles equal to 90°. More importantly, it's the shape of a rectangular pizza.
A parallelogram with all angles equal to 90°.
A parallelogram with four right angles. Also known as "The Equiangulizer" because any equiangular quadrilateral is automatically a rectangle.
A quadrilateral with four right angles.
A quadrilateral with four right angles. These guys have a pretty simple area formula:
A =
lw, where
l is the length and
w is the width. It doesn't get much more chill than that.
Regular Polygon
an equilateral, equiangular polygon
A shape whose sides are all equal in length and whose angles are all equal in measure.
These are polygons that have all equal sides and angles. A stop sign is an example of a regular octagon.
A shape whose sides are all equal in length and whose angles are all equal in measure.
Polygons where all the angles and sides are equal. Squares, equilateral triangles, and stop signs are all regular polygons we've met a time or two before.
Regular Prism
a prism with rectangular bases, six faces, all right angles and parallel opposite faces
A prism with bases that are regular polygons. Also, a prism that has daily scheduled trips to the potty.
Rhombus
a quadrilateral with parallel opposite sides, congruent opposite angles, supplementary adjacent angles and four congruent sides; a square after running a marathon might tilt like a rhombus
A quadrilateral whose four sides are all equilateral. Rhombi (that's the plural of rhombus) have all the properties of parallelograms, too.
A quadrilateral with four sides of equal length. So actually, rhombi are a girl's best friend.
A fancy-shmancy type of parallelogram with four equal sides and perpendicular diagonals. We can either use our trusty parallelogram area formula,
A =
bh, or the slightly cooler diagonal area formula,
A = ½
d1d2. It just depends on what the problem gives us.
Right Angle
an angle that is exactly 90°; often seen with a small box in the corner
An angle that measures exactly 90°. Naturally, that means any angle that doesn't measure 90° is wrong.
A 90° angle. Of course, that makes any angle that doesn't have a 90° angle wrong.
An angle that measures exactly 90°. Naturally, that means any angle that doesn't measure 90° is wrong.Right Triangle
a triangle with one right angle (a 90° angle); can also be on the left side
A triangle that has an angle that's exactly 90°. Or possibly a triangle that's just never wrong about anything.
A triangle with one angle of exactly 90°. You can't argue with it, because it's always right. (Groan.)
A triangle with one right angle. Super important when trigonometry rolls around, but kind of just a really specific definition for now.
Roots
The
roots of a quadratic expression in
x are the values of
x that make the expression equal to 0.
opposites of powers; trees have roots as do numbers; in this case it can be the square (the number that multiplied by itself twice equals), the cube (multipled three times), or the nth (you get the picture)
The organs responsible for getting nutrients from the soil, among other things. Dig in the ground a little bit, and you’ll probably come across one of these pretty soon. You may even see some aboveground, if there are large trees growing near any of your sidewalks. Did you know that tree roots are the main cause of water pipe damage? Roots are surprisingly strong, and they don’t always grow underground, either.
The points where the whole polynomial is equal to zero. If a root is real (i.e. not an imaginary or complex number), it also tells us what the function's
x-intercepts are. When graphing a polynomial function, these things are the root of our solution.
Much like the support system of a mighty tree, the roots of words are the base on which affixes can be added to alter the word and make it anew. What once was a mere "agree" can transform into "disagree" with a prefix and a "disagreement" with an additional suffix. Beautiful.
Scalene Triangle
a triangle with all sides of different lengths
A triangle whose three sides are all different lengths. He's probably just going through a growth spurt.
A triangle with none of the side lengths being equal. It's every side for himself. Or herself—maybe you have a female scalene triangle.
Scatter Plots
a type of plot that shows individual data values; dog doing its business outside on a windy day might do this to the snow
A graph that sort of looks like someone has been chucking darts at it, except that all those dots are data points. These graphs are a good way to see if there any correlations in our data.
Scientific Notation
an operation using exponents to write very large and very small numbers. For example, the scientific notation for .00004 is 4 X 10^-5
A coefficient, a 10, and an exponent walk into a lab, and say "Let's make some science."
A process used to work with very large or very small numbers using exponents with the power of ten. The standard form is
M × 10
n.
A way to abbreviate both gigantic numbers and miniscule numbers. Our pencils thank us for not wasting their precious life blood on so many zeros.
Secant
a line intersecting a circle at two points
A line that intersects a circle at two points. Really, line, how intrusive can you get?
A line that intersects a circle at two points.
We get this when cosine goes topsy-turvy. The reciprocal of the cosine function or just the cosine function flipped over.
Septagon
a seven-sided figure
Side
the straight edge of a polygon
Significant Digit
all non-zero digits in a number
Similar Figures
two figures that have the same shape, but not the same size; siblings - one exercises and one eats doughnuts
Figures that are proportional to one another and have the same angles. The only difference is their relative sizes.
Figures that share the same angle measures, and their sides lengths are all proportional. A fake mustache won't really disguise the fact that they are so similar.
Figures that are proportional to one another and have the same angles. The only difference is their relative sizes, and their taste in bolivian orchestras.
Slope
The steepness of a line, calculated as rise over run; think skiing (the bunny slope is less steep than the triple black diamond).
The measure of a line's steepness.
The steepness of a line, calculated as rise over run; think skiing (the bunny slope is less steep than the triple black diamond).
The "steepness" of a line. On the coordinate plane, it's calculated as "rise over run," or the vertical difference between two points divided by the horizontal difference between those same points.
The steepness of the line as you move along from left to right.
How steep a line is. It's measured as rise over run. Think ski slopes.
The difference between black diamond and the bunny hill. The measure of a line's steepness.
Measures the steepness of a line. Constantly rises to the occasion and runs over the competition.
A number that summarizes the rate of change of a line (also known as rise over run). It tells us whether the ski hill made by our line would be a green circle or a black diamond.
The steepness of the line as it moves along from left to right. Try not to fall off of it. It's a long way down.
The change in the
y-axis over the change in the
x-axis, or (
y2 –
y1)/(
x2 –
x1).
The steepness of the graph at a given point. Slope is defined as the vertical change between two ordered pairs divided by the horizontal change between those pairs.
The ratio of a line's changes in
y over its changes in
x.
The steepness of the graph at a given point. Slope is defined as the vertical change between two ordered pairs divided by the horizontal change between those pairs, or change in
y over change in
x.
Slope is how fast a line on a graph is going up or down. The formula for slope is: slope =
Δy/
Δx. You can remember that the
y goes on the top because it only has three legs, where
x has four, so
y is lighter and floats to the top.
Slope is how fast a line on a graph is going up or down. The formula for slope is: slope =
Δy/
Δx. You can remember that the
y goes on the top because it only has three legs, where
x has four, so
y is lighter and floats to the top.
Slope-Intercept Form
a representation of the equation of line in y = mx + b form, where m is the slope and b is the y-intercept
The special way true mathematicians communicate linear equations to each other. The format is
y =
mx +
b, where
m is the slope and
b is the
y-intercept of the line.
The equation of a linear function in the form
y =
mx +
b, where
m is the slope and
b is the
y-intercept of the line. Some mathematicians play favorites and like this form the best.
The slope-intercept form of a line is
y =
mx +
b, where
m is the slope and
b is the
y-intercept.
For a line with slope
m and
y-intercept
b, the line's slope-intercept form is:
y =
mx +
b.
Sloth
Laziness; inactivity; sluggishness
Sphere
a solid figure where all points are an equal distance from the center point; a ball
A ball. It's a central point that includes all the points a certain distance away from it in space. It's like a circle—but in 3D.
A solid in which all points are an equal distance from a central point. In other words, a ball.
No, you haven't stumbled into a geometry course. A sphere is a planet controlled by an angelic intelligence. In metaphors, "sphere" can also refer to the human body.
A ball-shaped figure in which every surface point is equidistant from a center. A keystone of sports everywhere.
Square
A parallelogram with all angles equal to 90° and all sides equal. Read: glorified rectangle.
A parallelogram with all angles equal to 90° and all sides equal in length.
A number raised to the power of two.
A rectangle with four congruent sides. It's also got that 90° angles thing going on plus the bisected congruent perpendicular diagonals. It's also the quadrilateral worthy of the title "regular."
A quadrilateral with four right angles and four sides of equal length.
A quadrilateral with four right angles and four equal side lengths. It's technically a special type of rectangle, so we use a modified version of the rectangle area formula. The length and width are identical twins (fun identical, not creepy-horror-movie identical), so a square's area is just
A =
s2, where
s is the side length.
Square Pyramid
a pyramid with a square base; all sides on the bottom are the same and the top looks like a place in Egypt
Standard Form Of A Line
a representation of a line in Ax + By = C form, where A, B, and C are all integers and A is also positive
Statistics
the branch of math that deals with collecting and analyzing data; you can justify almost anything with the right stats
Statistics is a way to lie to people or, at the very least, confuse them enough about a topic with have the math backing us up so that it makes us sound like we know what we’re talking about. At least that’s how unscrupulous people who wants to push an agenda define statistics. Instead, statistics is the study of patterns in a set of data and how those patterns can be interpreted or displayed to help us gain an understanding of what the data really represents. Speaking of patterns in data, how about Abraham Wald’s Memo from WWII?
Seems the Allies were trying to analyze the damage to planes coming back from missions over Germany to see if they could find a pattern in the damage and therefore armor against that damage pattern. Wald’s statistical analysis of the damage pattern on planes led them to armor specific areas of the planes and greatly, like saved countless lives greatly, improved the durability of the planes. Want some statistical weirdness? There’s nearly a perfectly linear relationship between per capita cheese consumption and the number of people who die by becoming tangled in their bedsheets. What about when statistics sent an innocent women to jail?
Lucia de Berk, a nurse, was sentenced to jail for the murder and attempted murder of children in the hospital where she worked based solely on the fact that there was a 1 in 342 million chance that one nurse’s schedule could randomly have lined up to have that nurse working on the nights of all the crimes. Lucia had to have been the culprit because it was so statistically unlikely for there to be another person with the same access to the victims. There was no physical evidence nor eye-witness testimony. She was later cleared when the real culprit was identified and confessed. Just so we’re clear, no decent court should ever convict someone solely on statistical evidence.
The math of taking a bunch of data and turning it into values that allow us to conclude something about that data.
Straight Angle
180° angle; basically a straight line
An angle that measures 180°. The title a straight line gives itself when it wants to seem more impressive.
A 180° angle. Or a straight line with an identity crisis.
A 180° angle. Or a straight line with an identity crisis.
Supplementary Angles
angles that add up to 180°; think a flat line when the angles are put together
Two angles that add up to 180°. It doesn't matter if they're adjacent or on different planets.
Two angles that add up to 180°.
Surface Area
the total area of all faces of a 3-dimensional object
The total 2D area on the outside of a 3D solid, a.k.a. the amount of gift-wrapping paper it takes to cover all of particular shape. You can use newspaper instead of gift-wrapping paper; it's a lot cheaper.
The two-dimensional area needed to cover the entire figure perfectly with no gaps or overlaps.
The area of all of the space figure's surfaces combined.
The total exterior surface of a solid. If we took our shape to the beach, it's everywhere it would get sand.
A measure of the amount of 2D space it takes to cover a 3D figure without gaps or overlaps.
The measure of the total area of all of a 3D shape's faces.
A measure of the outside surface of a three-dimensional object. Cells have surface area, and a large surface area is a very good thing to have.
Tangent
a line intersecting a circle at exactly one point
A line that intersects a circle at exactly one point (the point of tangency). The word "tangent" literally means "touching." So a line tangent to a circle is "just touching" the circle.
A line that intersects the circle at exactly one point. Or a polite man who's been in the sun for a while.
The ratio of the side opposite the reference angle to the side adjacent to it in a right triangle.
In a right triangle, tangent equals an angle's opposite side over its adjacent side.
Term
each part of an expression separated by addition or subtraction
A collection of numbers and variables in an expression separated by a plus or minus sign. They can be as simple as 3 or as complicated as -934
a7b3.
The smallest mathematical unit separated by + or – signs. If the expression 7
x + 2
y – 17 +
z was a family, then 7
x, 2
y, -17, and
z would be the terms.
A piece of an expression that is added or subtracted. A term can be a variable, a constant, or the product of constants and/or variables.
One piece of an expression, separated by the other pieces by a + or – sign. A term can be a number, a variable, or a combination of numbers and/or variables.
Parts of algebraic expressions that are separated by an addition or subtraction sign. 3 +
x + 5
x2 has three terms. They are all those "Terms and Conditions" you agreed to without reading them coming back to haunt you.
The amount of time you have to repay a loan.
The longer the term, the less you will pay each month but the more you'll pay overall in interest on your loan.
Example
John takes out a 3-year, $50,000 car loan to help him pay for his new BMW. The term of his loan is 3 years.
Tetrahedron
a pyramid with a triangular base
Theoretical Probability
The probability of an event determined by favorable outcomes ÷ possible outcomes; what math predicts, not necessarily what really happens.
This is the number-crunching side of probability. Statisticians attempt to predict what might happen in the real world using math theories and numbers.
How likely an event is to occur. When all outcomes are equally likely, it's the number of favorable outcomes in the sample space divided by the number of possible outcomes.
Three Dimensional Solid Objects
objects with width, length and height; not just flat, think
AvatarTransversal
a line that intersects two or more lines
A line that intersects two other lines, forming a total of eight angles. If the other two lines are parallel (and they usually are), then all these angles are special in some way.
A line that cuts across two other lines.
A line that crosses two or more parallel lines.
A line which crosses over a pair of parallel lines to create eight angles. It's a classic third-wheel scenario.
A line that intersects two other lines, forming a total of eight angles. If the other two lines are parallel (and they usually are), then all these angles are special in so
A line that intersects two other lines, forming a total of eight angles. If the other two lines are parallel (and they usually are), then all these angles are special in some way.
Trapezoid
A four-sided, closed shape with straight lines and only one pair of opposite sides equal. The best of all shapes, because of how much fun it is to say "zoid."
A four-sided closed shape with straight lines and only one pair of opposite sides equal in length.
A quadrilateral with
only one set of parallel sides (called the "bases"). Trapezoids absolutely cannot have two sets of parallel sides. That's a big fat no-no.
A quadrilateral with only one pair of parallel sides. These guys look a little funky compared to the fancy shmancy parallelograms.
A quadrilateral with only one set of parallel sides. Trapezoids refuse to follow the crowd, and they've even got their own area formula:
A = ½(
b1 +
b2)
h, where
b1 and
b2 are the parallel bases and
h is the height.
Triangular Prism
a prism with triangle bases; only the bases are parallel
A prism with a triangular base.
Triangular Pyramid (aka Tetrahedron)
a pyramid with triangular base; a tetrahedron made up of four equilateral triangles is called a regular tetrahedron
Trinomial
an algebraic expression with three terms
A polynomial with three terms.
A polynomial with three terms. Third time's a charm.
Two-dimensional
flat objects and shapes; think of a piece of paper; these objects only have two of the following: width, length, or height
Unit Rate
Price per unit; written as a ratio (same as unit cost).
A ratio that compares two different units in which the second number is 1. Whacking 144 moles in 3 minutes is a ratio, but whacking 48 moles per minute is a unit rate (the ratio was reduced from 144:3 to 48:1).
A simplified ratio of two numbers with (usually different) units. Speed, density, and price per weight are common examples. If you say it, "something per something," it's probably a rate.
In a given situation, the ratio between the value of
y and
x. Looks a lot like the slope if you squint at it.
A ratio relating things of two different types. Unit rate tells us how much of Thing A corresponds to one unit of Thing B—such as how many miles our Hummer gets per gallon of gasoline.
Vertex
The point where two rays meet; the corner of a polygon (plural is vertices).
The single maximum or minimum value of a parabola.
A point on a 3D figure where two or more edges meet.
The point at an intersection of two lines. It usually refers to a specific point within a larger figure (like an angle or a shape).
The sharp, pointy bit of an absolute value graph.
The point at which a parabola changes from increasing to decreasing or vice versa. Also where the slope is equal to zero. It's where things start looking up (or down). It's located at (
h,
k) if the equation is in vertex form.
In conic sections, the point (or points) on a curve where the graph changes direction; sometimes a maximum or minimum.
The point at an intersection of two lines. It usually refers to a specific point within a larger figure (like an angle or a shape).
The corner where two sides of a polygon meet, or where 3 or more faces of a 3D object meet.
Vertical Angles
when two lines intersect, opposite angles are called "vertical angles", these angles are congruent
Angles that are opposite each other resulting from two intersecting lines. Vertical angles are
always congruent.
A pair of angles opposite one another formed by the intersection of two lines. Oh, and they're congruent, too.
Angles formed when two lines cross. They lie across the vertex from one another and are always congruent. It's like a pair of twins sitting across from one another in a booth and sharing a milkshake.
Volume
the amount of space inside a 3-dimensional object.
The amount of three-dimensional space that an object takes up. Or what your mom asks you to turn down when you're listening to "that noise you kids call music nowadays."
The amount of 1 × 1 × 1 unit cubes that would fit inside a solid. In other words, it's a measure of how much 3D space a solid takes up.
The amount of space a space figure takes up. It's also how much soda fits inside a soda can.
A measurement of the amount of space contained within a three-dimensional shape.
The amount of space that an object occupies or encloses. If the object was our pants pocket, its volume is how much candy we can smuggle into the movie theatre.
The space inside a 3D object, like a cereal bowl or your brother's head. Insert your own joke here.
The measure of the amount of space inside a 3D shape.
The amount of space a solid takes up. It's also how much soda fits inside a soda can.
As in the number of shares trading. Not dialing up the woofers to 11.
As applied to trading, big volume increases are usually a bullish sign: more money coming into the market, more people engaged in the capital markets dance, louder music on the dance floor.
As in the number of shares trading. Not dialing up the woofers to 11.
As applied to trading, big volume increases are usually a bullish sign: more money coming into the market, more people engaged in the capital markets dance, louder music on the dance floor.
A measure of the stuff inside a three-dimensional object. Cells like to keep their volumes small, relative to their surface areas, so that matter and energy can be diffused into the cell efficiently.
X- Axis
the horizontal axis on a coordinate graph
Y- Axis
the vertical axis on a coordinate graph
 © 2024 Shmoop University, Inc. All rights reserved.
© 2024 Shmoop University, Inc. All rights reserved.


 )
)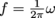 . Frequency—normal frequency, not angular frequency—is the inverse of the period of the wave:
. Frequency—normal frequency, not angular frequency—is the inverse of the period of the wave: 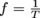 . The frequency of a wave is also inversely related to the wave's wavelength:
. The frequency of a wave is also inversely related to the wave's wavelength:  . Higher frequency light has more energy than lower frequency light, and looks more blue than red. Higher frequency sound is higher in pitch than lower frequency sound. Higher frequency flyer miles can get you a better seat on selected domestic flights.
. Higher frequency light has more energy than lower frequency light, and looks more blue than red. Higher frequency sound is higher in pitch than lower frequency sound. Higher frequency flyer miles can get you a better seat on selected domestic flights.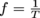 . Higher frequency light has more energy than lower frequency light, and looks more blue than red. Higher frequency sound is higher in pitch than lower frequency sound. Higher frequency flyer miles can get you a better seat on selected domestic flights.
. Higher frequency light has more energy than lower frequency light, and looks more blue than red. Higher frequency sound is higher in pitch than lower frequency sound. Higher frequency flyer miles can get you a better seat on selected domestic flights.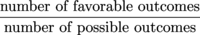
 ), with the word "to" in between (3 to 5), or with a colon (9:2). Not to be confused with CSI: Miami's Horatio.
), with the word "to" in between (3 to 5), or with a colon (9:2). Not to be confused with CSI: Miami's Horatio.