Example 1
Let R be the region between the graph of f(x) = x2+ 1 and the x-axis on the interval [0, 4].
- Draw R and the 8 rectangles that result from using a right-hand sum with 8 sub-intervals to approximate the area of R.
- Use a Right-Hand Sum with 8 sub-intervals to approximate the area of R.
- Is your approximation an under-estimate or an over-estimate to the actual area of R?
Example 2
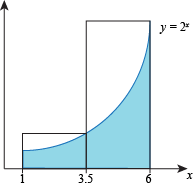
Let S be the area between the graph of y = f (x) = 2x and the x-axis on the interval [1, 6].
- Draw S.
- Use a Right-Hand Sum with 2 subintervals to approximate the area of S. Draw S and the rectangles used in this Right-Hand Sum on the same graph.
- Use a Right-Hand Sum with 5 subintervals to approximate the area of S. Draw S and the rectangles used in this Right-Hand Sum on the same graph.
- Are your approximations in parts bigger or smaller than the actual area of S?
Example 3
Let W be the area between the graph of  and the x-axis on the interval [1, 4].
and the x-axis on the interval [1, 4].
- Draw W.
- Use a Right-Hand Sum with 3 subintervals to approximate the area of W. Draw W and the rectangles used in this Right-Hand Sum on the same graph.
- Use a Right-Hand Sum with 6 subintervals to approximate the area of W. Draw W and the rectangles used in this Right-Hand Sum on the same graph.
- Are your approximations bigger or smaller than the actual area of W?
Example 4
The table below shows some values of the increasing function f(x).

- Use a right-hand sum with one sub-interval to estimate the area between the graph of f and the x-axis on the interval [2, 8].
- Use a right-hand sum with three sub-intervals to estimate the area between the graph of f and the x-axis on the interval [2, 8].
- Are your answers over- or under-estimates of the actual area between the graph of f and the x-axis on the interval [2, 8]?
Example 5
Some values of the decreasing function g are given in the table below:

- Use a right-hand sum with 3 sub-intervals to estimate the area between the graph of g and the x-axis on the interval [-1, 2].
- Use a right-hand sum with 2 sub-intervals to estimate the area between the graph of g and the x-axis on the interval [-1, 2].
- Are your answers over- or under-estimates for the actual area between the graph of g and the x-axis on the interval [-1, 2]?
Example 6
- Let W be the region between the graph of f and the x-axis on the interval [-20, 20].

Use a right-hand sum with 4 sub-intervals to estimate the area of W.
Example 7
- Let Z be the region between the graph of g and the x-axis on the interval [-4, 0].

- Use a right-hand sum with 2 sub-intervals to estimate the area of Z.
- Use a right-hand sum with 4 sub-intervals to estimate the area of Z.
- Are your answers over- or under- estimates for the area of Z?
Example 8
Let f(x) = x2 + 6x + 9. Use a right-hand sum with 6 sub-intervals to estimate the area between the graph of f and the x-axis on the interval [-6,-3].
Example 9
Let f (x) = -x2 + 2x + 8. Use a right-hand sum with 8 sub-intervals to estimate the area between the graph of f and the x-axis on the interval [0, 4].
Example 10
Let g be a function with values given by the table below. Use a right-hand sum with 3 sub-intervals to estimate the area between the graph of g and the x-axis on the interval [0, 12].

Example 11
Let h be a function with values given by the table below. Use a right-hand sum with 9 sub-intervals to estimate the area between the graph of h and the x-axis on the interval [-9, 9].

Example 12
The function f (x) on the interval [0, 30] is graphed below. Use a right-hand sum with 3 sub-intervals to estimate the area between the graph of f and the x-axis on this interval.

Example 13
Use a right-hand sum with the sub-intervals indicated by the data in the table to estimate the area between the graph of f and the x-axis on the interval [-10,1].





 .
.








 .
.
 .
.










 .
.













