The Fundamental Theorem of Calculus Introduction

The Fundamental Theorem of Calculus—or FTC if you're texting your BFF about said theorem—proves that derivatives are the yin to integral's yang. It's what makes these inverse operations join hands and skip. We thought they didn't get along, always wanting to do the opposite thing. All they needed was a little TLC from the FTC. Who knew?

The Fundamental Theorem of Calculus is what officially shows how integrals and derivatives are linked to one another. The FTC is super important—dare we say integral—when learning about definite and indefinite integrals, so give it some love. It's also one of the theorems that pops up on exams. Just saying.

To start with a realistic example, we'll check out what it means to integrate the velocity function. When velocity is constant, let's say we're driving due west at 65 MPH in search of a Sheetz MTO (or perhaps you prefer Wawa), we can find distance travelled using the formula

distance travelled = speed × time.

When the velocity v(t) is changing we can find the distance travelled using an integral instead:

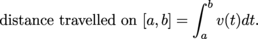
Generalizing to other types of functions we get the first Fundamental Theorem of Calculus, which says we can find the change in f on an interval by integrating f's rate of change:

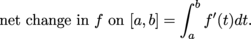
The first Fundamental Theorem of Calculus also finally lets us exactly evaluate (instead of approximate) integrals like

.

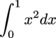There's also a second Fundamental Theorem of Calculus that tells us how to build functions with particular derivatives. We won't necessarily have nice formulas for these functions, but that's okay–we can deal.