To Be a Reactant or a Product, That is the Question

So far we've covered all of the basic details that are necessary to understand stoichiometry. The question remains, however. What is stoichiometry, and what does it have to do with molecular weights, molar masses, reactants, and products? 

Now that we have a basic understanding about the relationship between atoms, molecules, moles, and more, we can start understanding exactly how much reactant is consumed in a reaction and exactly how much product is formed. This is the basis of stoichiometry…and the vast majority of exam and quiz questions.



A basic question often raised in a chemical laboratory (or on an exam) is, "How much of product B will be formed from reacting specific amounts of reactant A?" Or the reverse question might be asked, "How much starting material A is needed to obtain a specific amount of product B?" We're here to show you how to solve these types of questions. Whip out your tool belt, and grab the instrument labeled stoichiometry. It is time to get quantitative.

It doesn't matter if the units given for reactants (or products) are in moles, grams, liters, or any other units imaginable. The key to solving stoichiometry problems is to first convert any given unit to moles. Repeat: always convert to moles. From there we use an approach called the mole method, which simply means that the coefficients in a chemical equation can be interpreted as the number of moles of each substance. An example will clear up any confusion. Consider the following equation:

N₂ + 3H₂ → 2NH₃

The mole method tells us that 1 mole of N₂ reacts with 3 moles of H₂ to give 2 moles of NH₃. The coefficients are vital in computing stoichiometry questions accurately. That's why it's so important to have a balanced equation. Good thing we're experts. (Basically.)

Let's start off with a simple example using the same above equation. How many moles of NH₃ are produced when 6 moles of H₂ is reacted completely with N₂?

We first need an essential conversion factor that we can effortlessly get from our equation. For every 3 moles of H₂ consumed, 2 moles of NH₃ is produced. Here is our conversion factor in equation form.



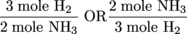
You may be wondering why we showed two conversion factors. The truth is we didn't. Both conversion factors mean the same thing except we have to choose the right one that will help cancel out units in the problem. The take home lesson is: Don't be afraid to throw conversion factors upside down as long as you also reverse the units. Back to the problem at hand:



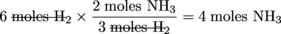
To solve the problem we just started with the 6 moles and used the appropriate conversion factor to give us our answer. The conversion factor we chose allowed us to cancel the units of moles H₂.
Now suppose the question was changed a bit: How many grams of NH₃ is produced when 16.0 g H₂ is reacted completely with N₂?

We've already learned in previous sections about the relationship between grams and moles. The key to solving this problem is to convert the given gram quantity into moles, use a conversion factor to get moles of NH₃ produced, and then convert that mole quantity into grams. Again, notice that even though our final answer is in grams, we will still use the mole method. There is no way of getting around the mole in chemistry.

grams of H₂ → moles of H₂ → moles of NH₃→ grams of NH₃



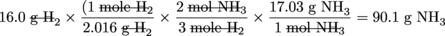
Just remember that moles are friendly little guys. If you can comfortably convert to moles and convert moles to other units, you're in business.

Brain Snack

What happens when Avatar and chemistry unite?