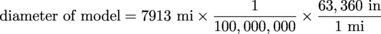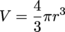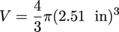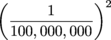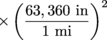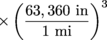Congruent and Similar Solids Examples

Are the solids similar, congruent, or neither?

They're both right triangular pyramids. That's good place to start. We can compare their base legs and hypotenuses and slant heights to see if they're similar or not.

They're all ratios of 0.2 or ⅕, which is our scale factor. That means the two triangular pyramids are similar.

Are the solids similar, congruent, or neither?

The two solids are right cones, each with a radius of 4 meters. That means they'll either be congruent or not at all similar, depending on the heights (both slant and normal).

We'll calculate the slant height of the first cone. How? In a world ravaged by right angles, Pythagoras is our only hope.

a² + b² = c²
(4 m)² + (7 m)² = c²
c ≈ 8.06 m

They're identical. If we calculate the normal height for the second cone, it'll end up being 7 meters. Also identical. Cone-incidence? We think not. Cone-gruence? Yes, indeed.

You're making a Styrofoam scale model of the Earth for your astronomy class. It's going to be totally far-out. If the diameter of the Earth is 7913 miles and you want your model to be one hundred million times smaller, what would be the radius, surface area, and volume of your model? There are 63,360 inches in a mile.

If there are 63,360 inches in a mile, we can do a quick calculation and find out how long the model's diameter is supposed to be. First, reduce the diameter of the Earth by the scale factor (not via shrink-ray) and then convert to inches.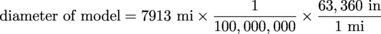

diameter of model ≈ 5.02 in

From there, we can find everything we need to know about the scale model. The radius is half the diameter (2.51 in), which we can use to find the surface area.

SA = 4πr²
SA = 4π(2.51 in)²
SA ≈ 79.2 in²

And the volume, too.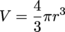

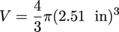
V ≈ 66.2 in³

We've found our measurements. To be completely, absolutely, positively, 100% certain that we have the right values, the best thing to do would be to check them using the surface area and volume ratios.

The surface area of the Earth would equal:

SA = 4πr²
SA = 4π(3956.5 mi)²
SA ≈ 196,712,612 mi²

If we want to find out the surface area of our scale model using that number, we have to make sure to square the scale factor and the miles-to-inches ratio.

SA of model = 196,712,612 mi² ×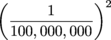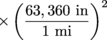
SA of model ≈ 79 in²

That's close enough. What about volume?

V = ⁴⁄₃πr³
V = ⁴⁄₃π(3956.5 mi)³
V ≈ 259,431,149,227 mi³

And now for some epic cubic unit conversion.

V of model = 259,431,149,227 mi³ ×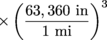
V of model ≈ 66 in³

Also pretty darn close. Looks like we did our work right, and your model of Earth is going to be stellar. Actually it'll be planetary, but that's more than Pluto can say.