Surface Area of Pyramids Examples

Find the lateral and surface area of the pyramid.

To find the lateral surface area, we need to know the perimeter of the base and the slant height. The perimeter is 0.8 × 6 = 4.8, but we need to futz around a bit to find the slant height.

If we split the hexagonal base into six triangles and then bisect them, we'll end up with twelve identical right triangles. The angles facing the center of the hexagon are . That means we have a 30-60-90 triangle with the shortest side equaling 0.4 inches (half of the hexagon's side), the hypotenuse equaling 0.8 inches (twice that), and the long side equaling . This might seem pointless, but just go with us.

If we look right at the pyramid instead of down onto it, we see that the altitude and the  side (perpendicular to the hexagon edge) create a right triangle with the slant height as the hypotenuse. We can smell the Pythagorean Theorem from a mile away.

a² + b² = c²

Substitute in our values. The two legs are the altitude (3 inches) and the distance from the center to the side ( inches). The slant height is the hypotenuse.

l² = 9 + (0.16 × 3)
l ≈ 3.08 in²

With the slant height, now we can find the lateral area. We use ½ in the lateral area formula for pyramids because pyramids halve triangles.

That's lateral area. Surface area is just the lateral area plus the area of the base. Since it's a hexagon, the base is going to be a bit tricky. We might not know hexagons that well (nothing against them), but triangles have been with us since the dawn of time. Six triangles have ½ base times height? We know the deal.

B ≈ 1.7 in²

Put 'em together and what have you got? Bippity boppity boo!

SA = L + B
SA = 7.4 in² + 1.7 in²
SA = 9.1 in²

Find the surface area of the entire figure.

This figure combines our knowledge of the surface area of pyramids and prisms. Oooh, fancy.

First, let's figure out exactly what we want. The surface area of the overall figure consists of the lateral area of the pyramid and the lateral area of the prism along with one base (the bottom). We'll say the formula looks like this:

SA = L_Pyramid + L_Prism + B

Okay…but what do these things even mean? We'll start with the lateral area of the prism. Like we said in the last chapter, it's a folded rectangle where the length is the perimeter of the base and the height is...the height. The perimeter is 15 + 15 + 15 + 15 = 60 ft and the height is 20 ft.

L_Prism = Ph
L_Prism = (60 ft)(20 ft) = 1200 ft²

Moving on to the lateral area of the pyramid. Pyramids halve triangles, remember?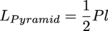

The perimeter is the same, but l is the slant height, which is 15 ft.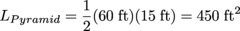

Two thirds done already? That's awesome.

Trivia Time: Do we have to find the base of the prism or of the pyramid? It's actually a trick question because they're both the same. The base is a square no matter how you look at it.

B = s²
B = (15 ft)²
B = 225 ft²

We have all the parts. All that's left is putting them together.

SA = L_Pyramid + L_Prism + B
SA = 450 ft² + 1200 ft² + 225 ft²
SA = 1875 ft²