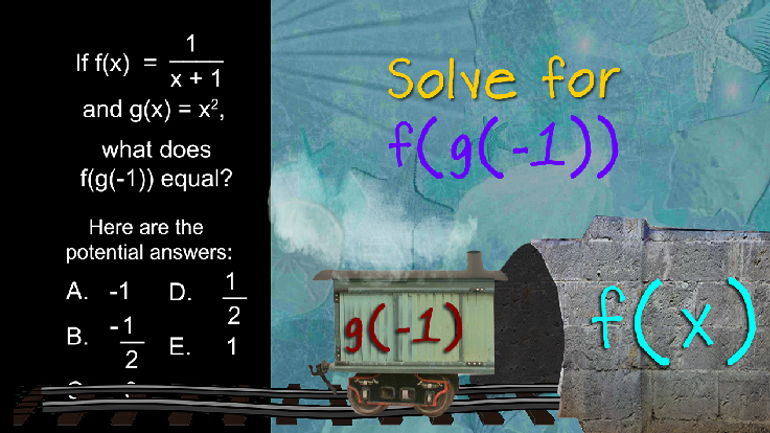ShmoopTube
Where Monty Python meets your 10th grade teacher.
Search Thousands of Shmoop Videos
Intermediate Algebra Videos 25 videos
ACT Math Intermediate Algebra Drill 1, Problem 1. What is the product of (a – 3)2?
ACT Math Intermediate Algebra Drill 1, Problem 2. Find the product of (2a + 6)2.
ACT Math Intermediate Algebra Drill 1, Problem 4. Solve for x based on the equation.
ACT Math 1.4 Intermediate Algebra 461 Views
Share It!
Description:
ACT Math Intermediate Algebra Drill 1, Problem 4. Solve for x based on the equation.
Transcript
- 00:02
And here is a shmoop question...
- 00:04
Solve: x2 + 6x = 0
- 00:08
And here are the potential answers...
- 00:13
Okay Just looking at the problem, it should cry out to us that it's a factor problem.
- 00:17
And we know we can factor an x out of there
Full Transcript
- 00:20
So... let's do it.
- 00:21
We have x times the quantity x plus 6.
- 00:23
So... x has to equal at least 0 here because 0 squared is 0 and 6 times 0 is... also zero.
- 00:31
But what else can x be? Well, we have this quantity here; x plus 6.
- 00:34
So... if x was NEGATIVE 6, this piece would be zero... and zero times anything is zero...
- 00:40
so negative 6 works as well.
- 00:42
...and our answer is D.
Related Videos
ACT Math: Plane Geometry Drill 3, Problem 1. What is the area of the trapezoid shape in the video?
ACT Math: Coordinate Geometry Drill 1, Problem 1. Which inequality is expressed by the number line?
ACT Math: Intermediate Algebra: Drill 3, Problem 1. Find the fifth number in the series.
We don't like knocking people down to size, but we do like simplifying radicals. Join us?
If fleeing criminals always fled the scene of the crime at perfect right angles, it would be much easier to determine their whereabouts. Fortunatel...