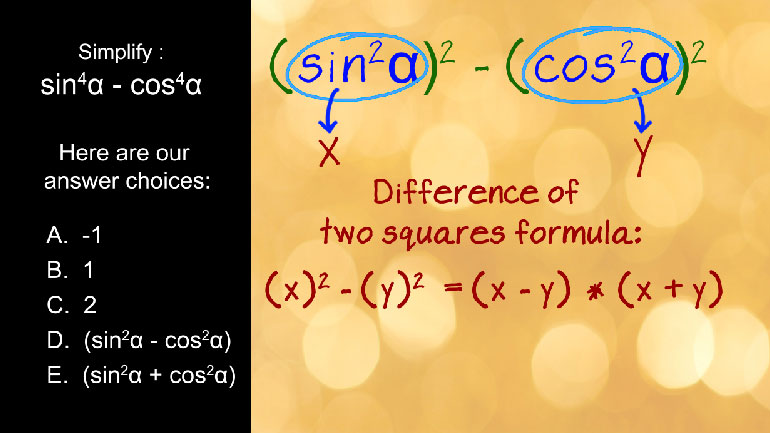ShmoopTube
Where Monty Python meets your 10th grade teacher.
Search Thousands of Shmoop Videos
Trig identities Videos 7 videos
ACT Math Trigonometry Drill 1, Problem 2. Can you find the tangent?
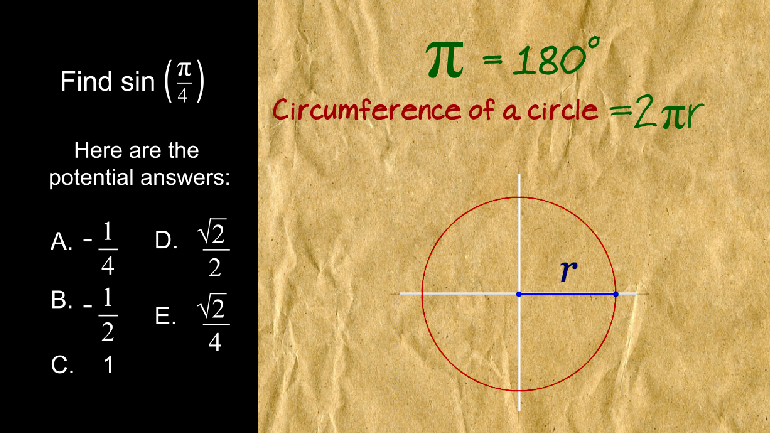
ACT Math: Trigonometry Drill 1, Problem 4. Can you find the sine?
ACT Math: Trigonometry Drill 1, Problem 3. Can you find the cosine?
ACT Math 4.1 Trigonometry 228 Views
Share It!
Description:
ACT Math: Trigonometry Drill 4, Problem 1. Simplify the expression.
Transcript
- 00:02
For a good time, solve this shmoopy question.
- 00:05
Simplify. sin4⍺ - cos4⍺ << sine to the fourth alpha minus cosine to the fourth alpha>>
- 00:10
And here are our answer choices...
- 00:16
Okay, the trick lies in recognizing the fact that 2 squared equals 4.
- 00:22
It means we can express sine to the fourth alpha minus cosine to
Full Transcript
- 00:26
the fourth alpha as sine squared alpha squared
- 00:29
minus cosine squared alpha squared... because they're the same thing.
- 00:33
Now we need to call on our good friend - the
- 00:35
difference of two squares formula: x squared minus y squared equals x minus y times x plus y.
- 00:43
If we pretend that sin squared alpha is x
- 00:45
and cos squared alpha is y, we can simplify it to:
- 00:49
sin squared alpha minus cosine squared alpha times sin squared alpha PLUS cosine squared
- 00:55
alpha. We also know that (sin2⍺
- 00:59
+ cos2⍺) = 1 from the fundamental trig identities.
- 01:03
Now all we're left with is (sin2⍺ -- cos2⍺)...
- 01:07
...which happens to be answer D.
Related Videos
ACT Math: Coordinate Geometry Drill 1, Problem 1. Which inequality is expressed by the number line?
ACT Math: Intermediate Algebra: Drill 3, Problem 1. Find the fifth number in the series.
ACT Math: Plane Geometry Drill 3, Problem 1. What is the area of the trapezoid shape in the video?
Haven't you always wondered how much cardboard it takes to encase a trunk warmer for your pet elephant?
Want to figure out the area and perimeter of irregular shapes? Break them down into regular shapes. For example, a flower can be broken down into s...