Example 1
Let R be the region above the line  and below the graph of y = cos x on the interval
and below the graph of y = cos x on the interval 
Write an integral expression for the area of R,
(a) vertical slices
(b) horizontal slices
Example 2
Let R be the region bounded by the curve y = x4 and the lines x = 2 and y = 81. Write an integral expression for the area of R, using
(a) vertical slices
(b) horizontal slices
Example 3
Let R be the region in the first quadrant bounded by the graph of x2 + y2 = 1 and the x- and y- axes. Write an integral expression for the area of R, using
(a) vertical slices
(b) horizontal slices
Example 4
Let R be the region bounded by the graphs of y = ex, y = 1, and x = 3.
Write an integral expression for the area of R, using
(a) vertical slices
(b) horizontal slices
Example 5
Let R be the region above the graph of y = 1 – x2 and below the graph x2 + y2 = 1. Write an integral expression for the area of R, using vertical slices.
Example 6
Let R be the region in the first quadrant bounded by the x-axis and the graphs  and y = 5 – x2. Write an integral expression for the area of R, using horizontal slices.
and y = 5 – x2. Write an integral expression for the area of R, using horizontal slices.
Example 7
Let R be the region in the first quadrant bounded by the line y = 5 and the graphs  and y = 5 – x2. Write an integral expression for the area of R, using horizontal slices.
and y = 5 – x2. Write an integral expression for the area of R, using horizontal slices.
Example 8
Let R be the region between the graphs  , y = x2, and the line x = 4.
, y = x2, and the line x = 4.
Write an integral expression for the area of R, using vertical slices.
Example 9
Let R be the region in the first quadrant bounded by the x-axis and the graphs  and y = 5 – x2. Write an integral expression for the area of R, using vertical slices.
and y = 5 – x2. Write an integral expression for the area of R, using vertical slices.
Example 10
Let R be the region in the first quadrant bounded by the line y = 5 and the graphs  and y = 5 – x2. Write an integral expression for the area of R, using vertical slices.
and y = 5 – x2. Write an integral expression for the area of R, using vertical slices.
Example 11
Let R be the region between the graphs of y = x4 + 1 and y = x2 + 1 for 1 ≤ y ≤ 5. Write an integral expression for the area of R, using
(a) vertical slices
(b) horizontal slices
Example 12
Let R be the region shown below.

Write an integral expression for the area of R, using vertical slices.
 :
:
 . This is the region R:
. This is the region R: on this interval, the height of the slice at position x is
on this interval, the height of the slice at position x is

 . The integral expression
. The integral expression


 to y = 1.
to y = 1.










 . Since y also ranges from 0 to 1, the area of R is
. Since y also ranges from 0 to 1, the area of R is













 (or x = 2y) on the left to the graph of y = 5 – x2 (or
(or x = 2y) on the left to the graph of y = 5 – x2 (or  ) on the right:
) on the right: , and the area of the slice is
, and the area of the slice is


 on the right.
on the right. , so the area of that slice is
, so the area of that slice is

 :
:


 , the line x = 2, and the x-axis. The piece on the right is bounded by the graph y = 5 – x2, the line x = 2, and the x-axis. Let's look at the piece on the left first. The slice at position x has height
, the line x = 2, and the x-axis. The piece on the right is bounded by the graph y = 5 – x2, the line x = 2, and the x-axis. Let's look at the piece on the left first. The slice at position x has height  , and x runs from 0 to 2. The area of the piece on the left is
, and x runs from 0 to 2. The area of the piece on the left is
 (since this is where the graph of 5 – x2 crosses the x-axis).
(since this is where the graph of 5 – x2 crosses the x-axis).

 .
.
 .
.

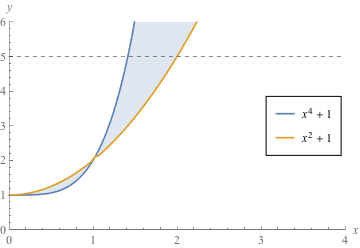
 . The graph y = x2 + 1 intersects the line y = 5 when x = 2.
. The graph y = x2 + 1 intersects the line y = 5 when x = 2. the graph of y = x4 + 1 is on top.
the graph of y = x4 + 1 is on top. the graph of y = 5 is on top.
the graph of y = 5 is on top.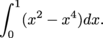
 .
.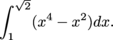
 .
.
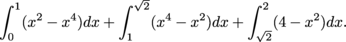
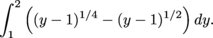


 immediately:
immediately:
 from
from  :
: ,
, .
. , because, for
, because, for  , the upper bound of R is a line, and for
, the upper bound of R is a line, and for  , the upper bound of R is the circle.
, the upper bound of R is the circle. the upper bound of R is a line that goes through the origin and has slope
the upper bound of R is a line that goes through the origin and has slope
 .
. ) is
) is

 in the left piece of R, the area of the left piece of R is
in the left piece of R, the area of the left piece of R is .
. the upper bound of R is the equation of the circle, x2 + y2 = 1 or
the upper bound of R is the equation of the circle, x2 + y2 = 1 or .
. ) is
) is

 to
to  in the right piece of R, the area of the right piece of R is
in the right piece of R, the area of the right piece of R is
