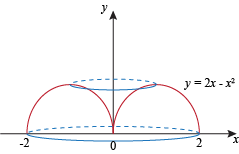
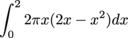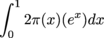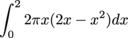Let R be the region bounded by the graph y = 2x – x2 and the x axis.
(a) Sketch the solid obtained by rotating R around the y-axis.
(b) Is it easier to use washers or shells to find the volume of the solid you drew in (a)? Why?
(c) Write an integral expression for the volume of the solid you drew in (a).
Answer
(a) The function y = 2x – x2 factors into
y = x(2 – x)
so this function hits the x-axis at x = 0 and x = 2.
(b) It's easier to use shells to find the volume of the solid. If we try to use washers, we run into a problem because the outside and inside radius are determined by the same function. Or because we don't know how to solve the equation
y = 2x – x2 for x.
Either way, washers aren't looking good. With shells, there aren't any such problems.
(c) In (b) we decided to use shells. Since the y-axis is the axis of rotation the shells need to run parallel to the y-axis.
The shell at position x has radius x and height y = 2x – x2, so the volume of the shell is
2πx(2x – x2) Δ x.
The shells go from x = 0 at the center of the solid to x = 2 at the outside of the solid. These are gonna be the limits of integration. The volume of the solid is