ShmoopTube
Where Monty Python meets your 10th grade teacher.
Search Thousands of Shmoop Videos
Area and Volume Videos 38 videos
Los cubos de hielo no son ruidosos generalmente...pero tienen un volumen de tipo diferente. Este video te enseñará cómo encontrar el volumen de...
Volume is crucial to our lives. It determines the amount of food we eat, the amount of fluid we drink, and hydraulics, just to to name a few. One o...
Surface Area of Cylinders 14741 Views
Share It!
Description:
Haven't you always wondered how much cardboard it takes to encase a trunk warmer for your pet elephant?
Transcript
- 00:05
Surface Area of Cylinders, a la Shmoop. You've adopted an elephant in Africa.
- 00:14
Normally you send a small amount of money every month to keep him fed, clothed, and
- 00:18
educated...
- 00:19
...but the holidays are coming up and you want to do something special... knit him a
- 00:25
trunk warmer. So it doesn't get damaged by the UPPS... the
Full Transcript
- 00:29
United Pachyderm Parcel Service... you need to ship it in a sturdy tube.
- 00:34
The trunk warmer will fit perfectly in a tube with radius three and height five.
- 00:39
You think you have enough cardboard to make the tube yourself. How much cardboard do you
- 00:44
need?
- 00:45
Here are your options: A cardboard shipping tube is a cylinder.
- 00:52
So to figure this problem out, we'll need to find the Surface Area of a Cylinder.
- 00:57
The finished product will look like this, but how do we find the area of it?
- 01:01
Just like you have a formula for knitting an elephant trunk warmer...
- 01:04
You can find anything on Pinterest...
- 01:07
There's a formula for finding the surface area of a cylinder.
- 01:10
But first, let's break down what the cylinder looks like when it's flattened...
- 01:14
Like it will be after your elephant sits on it.
- 01:16
First, we'll need to find the area of each of those circles.
- 01:20
The formula for finding the area of a circle is pi times the radius squared.
- 01:25
Our radius is three, so our formula will be pi times three squared.
- 01:30
Three squared is three times three, or nine.
- 01:34
Pi is approximately three-point-one-four, so three-point-one-four times nine is twenty-eight-point-two-six.
- 01:42
We have two circles, so we'll then need to double that number. Twenty-eight-point-two-six
- 01:50
times two is fifty-six-point-five-two.
- 01:56
This is easier than knitting that trunk warmer... it took forever to figure out what "purling"
- 02:00
means. But we still have to find the area of the
- 02:03
middle part of the cylindrical part of the cylinder...
- 02:06
... which is a rectangle when squashed flat. To find this area, we'll need to multiply
- 02:11
the height, which we know is five, by the circumference of the circle.
- 02:16
This makes up the top and bottom edge of the rectangle.
- 02:19
We can find out what this is by using the simple formula two pi "r."
- 02:24
Two times pi is approximately six-point-two-eight.
- 02:29
Six-point-two-eight times "r," which is three, is eighteen-point-eight-four.
- 02:35
Now we just multiply that by the height, five, to get the surface area of the middle part
- 02:40
of the cylinder, which is ninety-four-point-two. To get the total surface area, we just add
- 02:46
our two numbers together.
- 02:48
Fifty-six-point-five-two plus ninety-four-point-two equals one-hundred-fifty-point-seven-two.
- 02:55
So the answer is C.
- 02:57
Sounds like a lot of cardboard. Good thing you've bought quite a few nasal hair trimmers
- 03:01
over the years. The trunk warmer is knitted, the tube is made,
- 03:07
and off it goes.
- 03:08
We hope your elephant appreciates all the hard work you did.
Related Videos
Want to figure out the area and perimeter of irregular shapes? Break them down into regular shapes. For example, a flower can be broken down into s...
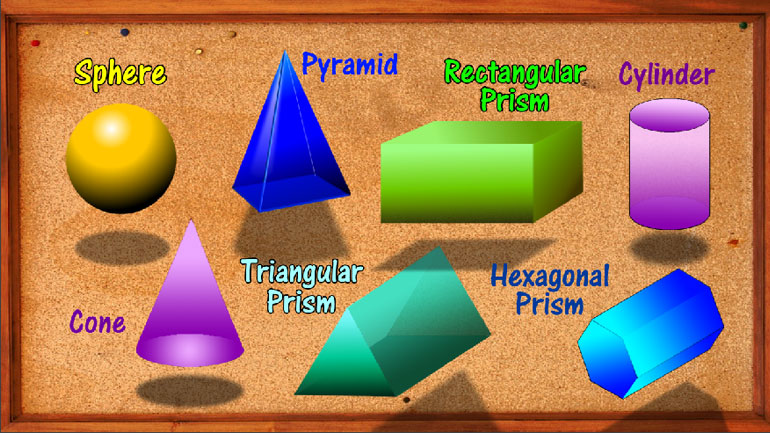
It's one thing when all those shapes are sitting flat on the page. But when they start popping out and invading our personal space bubble, we get a...
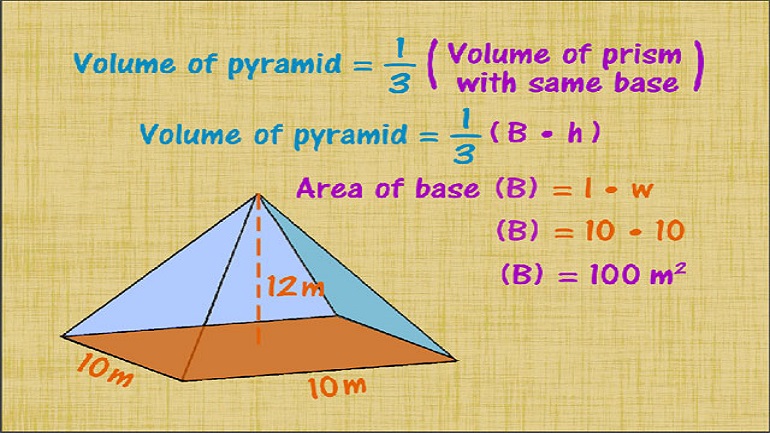
Does thinking about 3D Geometry get you bent out of shape? Never fear! Watch this video and figure out some fun new shapes to bend back into. We're...
ACT Math: Plane Geometry Drill 3, Problem 5. How long would it take for the wheel to make two rotations?