Limits at Infinity

What's the biggest thing you've ever seen? The world's largest rubber band ball? Maybe the ocean? Actually, the sun is pretty huge (understatement), and we see that every day. But then we could say that the sky covers pretty much the entire universe.

That seems hard to top. However, even that is puny compared to some limits, because they can go to infinity. We're talking about x as it gets really, really big or really, really small. This idea is known as the end behavior of a function, and that is what these limits at infinity will help us describe.

For the most part, these limits fall into three categories. Instead of wasting your time and ours, we'll just show you each one in a sample problem. After that, we'll cover a couple of complications and how to fix them, so you can really wow your friends at parties.

Sample Problem

Evaluate .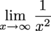

Hello again. Long time no see. But look at that limit; x isn't approaching some number, it's just going to go on and on and on forever. There are a couple ways to look at this. Let's look at its graph again:

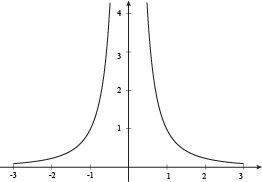
Notice what happens as x gets bigger and bigger. The y-values get closer and closer to zero. This means:

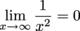
Another way to think about this is to consider what happens when we substitute really large values in for x. What if we used 1,000, for instance? We end up with a fraction of 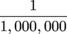. That's really small, a.k.a. close to zero. And it will only get smaller from there. Therefore:

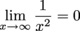
Our answers agree. Hurray. We hate when our answers fight.

There's a third way to find the limits at infinity, and it is even more useful. Whenever we are asked to evaluate the limit of a fraction, we should look at and compare the degree of the numerator and denominator. Like judges at a pompadour competition, we want to know which one is bigger.

For 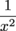, the bigger term is in the denominator. This means that as larger and larger numbers are put in for x, the denominator grows faster than the numerator. Okay, this is a bad example, because the numerator is constant, but you get the idea. The function is bottom heavy, which makes it sink to zero at infinity.

Sample Problem

Evaluate .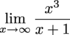

This time, we will skip right to checking the degree (although plugging in numbers will always work too). If the numerator is larger (like x³ is larger than x in this case), the function will head to infinity as x gets big. That means that:

The function will travel up and to the right, forever reaching towards the stars. You go, little function. Dream big.

This result makes makes perfect sense, because the numerator is going to get absolutely enormous while the denominator will get kind of big but not ridiculously so. Very exact, we know.

Sample Problem

Evaluate .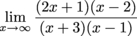

Now this limit is a little bit different from what we've seen in previous sections. You might be tempted to simplify and divide out and do crazy things like that. Fight the temptation, don't give in. Think about something else, like animals playing music. What we'll do instead is multiply things out, but only just a little bit.

Yes, you read that right; "who cares" and "doesn't matter" have now become official Shmoop limit lingo. All we need in order to evaluate this limit are the terms with of the largest exponent.

This time, the degree is the same, 2. Now we have a neck-and-neck race on our hands. It's going to be a photo phinish—er, finish—and it all comes down to the leading coefficient.

We have a 2 in the numerator (from 2x²), and a 1 down below (from x²). The variables essentially cancel out each other. Not literally, but only in terms of how the function approaches infinity. So, our limit will be:

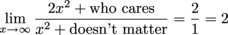
Don't believe us? Check out the graph here. There's a horizontal asymptote right at y = 2, which fits in perfectly with what we just found.

Sample Problem

Evaluate .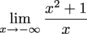

The degree of the numerator is larger than the degree of the denominator. Answer: ∞, right? Problem: done, right? Not so fast.

We have a limit as x approaches negative infinity this time. We aren't able to just throw that sideways crazy eight down and move on. Instead, we are forced to consider the sign of our final answer.

A square of anything will be positive, even something as super negative as -∞, so the numerator will be positive. The denominator, however, is going to be negative when you put in negative numbers for x.

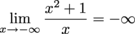
We have to watch the signs of our answers. A single minus sign can swing our answer from negative infinity to positive infinity. That's got to be the nastiest case of whiplash ever.

Sample Problem

Evaluate .

A quick glance might suggest that this function has x over x², and so the limit would be zero. Sorry, but the problem is weirder than that, because weird things are being done to our largest term. Here, the largest term in the denominator is square rooted. This means that the largest order cannot be thought of as 2 anymore, it's actually:

(x²)^1/2 = x^2/2 = x

Suddenly the numerator and denominator have the same order, forcing us to look at the coefficients. Just be careful with that square root. The edges are sharp.

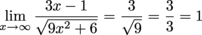
Yeah, we have to take the square root of 9 too. That gives us a limit of 1 as x approaches the endless void at the right side of the graph.

Sample Problem

Evaluate .

Okay, last problem time, and this one is a trig problem. We can't look at the degree of the function, so our previous method won't work. Try picturing sine's graph instead.

Maybe you are saying to yourself, "How will this limit work? When x goes to infinity, sine does this (you move your arm in a wavy motion in front of you) forever and ever and ever."

That hand movement does describe sine's behavior as x gets infinitely big. It goes back and fourth between 1 and -1 over, and over, and over again. So it doesn't really approach any value.

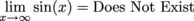
Sometimes there just isn't a limit. The function doesn't approach a single value, but it also doesn't get bigger or smaller without end. Why can't you just make up your mind, sine?

Summary

• If the degree on top of a fraction is smaller than the degree on bottom, it approaches zero.
• If the degree is larger up top, though, then the function approaches infinity.
• When the degrees are equal, we compare the leading coefficients. Their ratio is the horizontal asymptote that the function approaches.