Need for Speed 2: Electric Boogaloo

The song "Ten in the Bed" is a classic children's song. It also is completely insane. How do you get ten kids in a bed? And why is the little kid such a covers hog?

If you are scoring at home, the song goes something like this:

Verse	Kids in Bed	Kids on Floor
1	10	0
2	9	1
3	8	2
4	7	3
5	6	4

In the time it takes to sing each verse, we lose one kid in the bed and throw one kid on the floor. This is a little like an experiment used to determine a reaction rate. What is a reaction rate? Basically, it is the rate at which products form and reactants disappear in a reaction.

We can represent any reaction with this very basic equation:

Reactants → Products

This equation tells us that, during the course of a reaction, reactant molecules are consumed while product molecules are formed. The reaction rate is the change in concentration of the reactant (or the product) over time. To continue our super sweet analogy, if we think about our kids in the bed, our equation would look something like:

Kids in the Bed Before → Kids in the Bed After

Let's think about our most basic equation again:

A → B

As the number of A molecules decreases, the number of B molecules increases. Those super-smart super-nerdy scientists have come up with a very convenient way to express the reaction rate in terms of change in concentration with time. Need a visual? Check this out.



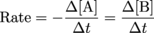
Need a translation? ∆[A] and ∆[B] are the changes in concentration (in molarity) over a period of ∆t. The ∆[A] is negative because the amount of A is decreasing. The ∆[B] is positive because the amount of B is increasing over time. In the simple A → B equation, the rate of formation of B is exactly opposite the rate of disappearance of A.

On the Double

What about a more complicated equation?

2A → B

How do you think our rates would change with this simple addition of a number 2? For the disappearance of 2 A molecules, 1 B molecule magically (or chemically) appears. Therefore:

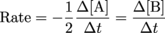
More Generally

Here's how we would put it into generic terms for a reaction with two reactants and two products:

aA + bB → cC + dD

The reaction rate is given by the amazing, chic, super cool equation:

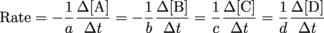
Get Real

Formulas are all fine and dandy, but how do scientists determine concentrations and rates in the real world? Good question. There are several ways scientists can measure these rates experimentally. In fact, they use a variety of instruments seen on CSI that conveniently save the day and solve the case every time.

One such instrument is a UV-Visible (UV-Vis) spectrophotometer, which can be used if a reaction involves a color change. There's also gas chromatography (GC). It can be used if the compounds are fairly volatile organic compounds. Other techniques include Nuclear magnetic resonance (NMR) spectroscopy or infrared spectroscopy (IR). These can be used to measure reaction rates for other organic compounds.

Above the Law

It's time to call the chemical police, because we've got some laws to break.

Okay, not really, but we should talk about rate laws. A rate law is an expression that relates the rate of a reaction to the rate constant and the concentration of the reactants. Hmmm, there's a lot going on in that sentence. Let's try and break it down.

First of all, a rate law is just an expression or a means of telling the reader something. In fact, it tells the reader how the rate relates to the change in concentration. The rate relates to the change in concentration via a fancy constant we already learned about—the rate constant, or k for short.

Let's just give it to you straight up.

aA + bB → cC + dD

For this reaction, the rate law looks like this:

rate = k [A]^x [B]^y

Where the heck did x and y come from? And where did C and D go? Let's explain further so no one thinks they're hallucinating. This rate law tells us that if we know the values of k, x, and y, as well as the concentrations of A and B, we can calculate the rate of the reaction.

Those sneaky x and y's are the orders of the reaction, and they're determined experimentally. While we're at it, we should also mention that k is determined experimentally as well. Sheesh, everything is so complicated and experimental in the world of chemistry.

How does somebody find all these values? Basically, a chemist will hold all conditions constant, and vary just the concentration of the reactants. Usually, one reactant's concentration is doubled at a time and the rate is checked.

Example Time? Example Time.

Let's give you an example based on the disturbing kids' song we heard earlier.

Kids in bed → Kids on floor
Rate of kids falling out of bed	# Kids in Bed
3 per minute	10
3 per minute	5

The kids fall out of bed at the same rate, regardless of whether there are "ten in the bed" or "five in the bed." There is no concentration effect on the rate. Nevertheless, we can use this information to derive a rate law equation that describes the reaction.

In the hypothetical kid dropping experiment, the rate law would look like this:

Rate = k[Kids in Bed]⁰

In the kids experiment, the rate equals the rate constant, k, multiplied by the concentration of the reactant (kids in bed) to the zero power (since there is zero change in the rate). Any number to the zero power is simply 1, so the rate is equal to k × 1 Or, y'know, just k.

A more generic rate law is given below to see how it works.

Real Example Time? Real Example Time.

Each reactant in a reaction is included in the rate law. The exponents for each concentration are determined experimentally. Here's some made-up data for a different reaction.

H2 (g) + Cl2 (g) → 2HCl (g)
Experiment	Initial H2 conc. (mol/L)	Initial Cl2 conc. (mol/L)	Initial Rate (mol/L*s)
1	1.00	1.00	2.3 × 10-5
2	2.00	1.00	4.6 × 10-5
3	1.00	2.00	9.2 × 10-5

As a reminder, for a reaction A + B → C + D, we have Rate = k[A]^x[B]^y.

The concentration of H₂ is doubled from experiment 1 to experiment 2, and the rate doubled as well. The concentration of Cl₂ doubled from experiment 1 to experiment 3, and the rate quadrupled. We can compare the ratio of two of these results at a time, and solve for each exponent that way.

First, let's check out the ratio of the Experiment 2 to Experiment 1. The only difference was that the concentration of H₂ was doubled; with this, we'll be able to track down the value for H₂'s exponent.



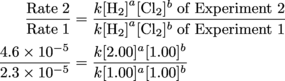
Heyo, k and [1.00]^b cancel out.



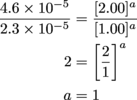
First exponent, down for the count. Now for Round 2; it'll have a bit less Experiment 2 in it, and a bit more Experiment 3.



Tada!

From these results, we can write the experimentally determined rate law:

Rate = k[H₂][Cl₂]²

So, Uh, What Does It All Mean?

The exponents in front of the concentration values in the rate law describe the reaction order of an experiment.

For example, in the kid-falling-out-of-bed experiment, the reaction is described as zeroth order (or 0th order). The second rate law can be described as first order (1st order) in H₂ and second order (2nd order) in Cl₂. The overall rate law for a reaction can be found by adding the exponents for each reactant in the rate law. So the overall rate law for the second reaction is 3rd order.

Common mistake alert! The stoichiometry of a reaction has nothing to do with the order of the reaction.

To see these concepts in action, check out this video on reaction rates for making "elephant toothpaste."

Brain Snack

Here are five absolutely hilarious science videos to tickle your funny bone.