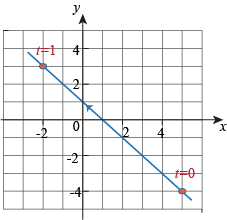
Example 1
What's a parametrization of
- the line that goes through the points (4, 8) and (10, 4), going from
- left to right.
- right to left.
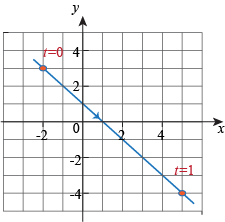
Example 2
Parametrize
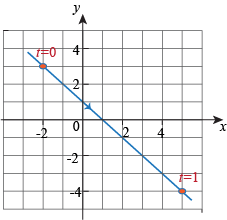
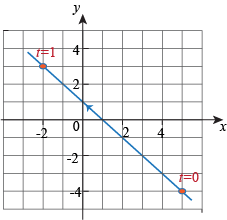
- The line that goes through the points (-2, 3) and (5, -4), going from
- left to right.
- right to left.
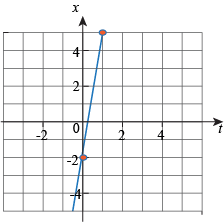
Example 3
Parametrize
- The line that takes 4 time steps to travel from (0, 5) to (20, 1).
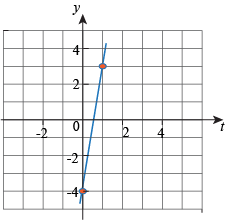
Example 4
Parametrize
- The line that takes 0.5 time steps to travel from (0, 5) to (20, 1).
Example 5
For the set of parametric equations, eliminate the parameter to find the equation of a line.
- x(t) = 5t and y(t) = t – 3
Example 6
For the set of parametric equations, eliminate the parameter to find the equation of a line.
- x(t) = 3t – 2 and y(t) = 6t + 1
Example 7
For the set of parametric equations, eliminate the parameter to find the equation of a line.
- x(t) = 4t + 1 and y(t) = 2t – 7









 ,
,



 the equation for x is
x(t) = 40t.
the equation for x is
x(t) = 40t. 
 so
the equation for y is
so
the equation for y is .
.

 .
.
 .
.