Points, Vectors, and Functions Exercises

Answer

These don't look like they have anything to do with each other!

• for 0 ≤ θ ≤ π

Answer

• for 0 ≤ θ ≤ 2π

Answer

• for 0 ≤ θ ≤ 4π.

Answer

Again, r is never negative because

r = 1 + sin θ

is always between 0 and 2.

Find the value of r at some nice angles.

This gives us some points to start from.

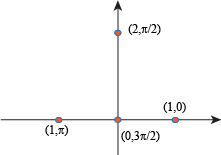
Now we need to figure out what's going on in between these points. Remember that r is never negative. 

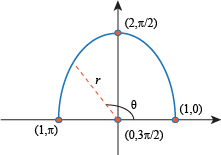
From θ = 0 to , the value of r will move from 1 to 2.

From to θ = π, the value of r will move from 2 to 1.

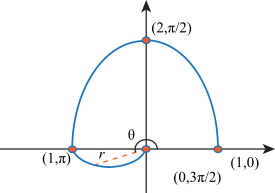
From θ = π to , the value of r will move from 1 to 0.

From to θ = 2π, the value of r will move from 0 to 1.

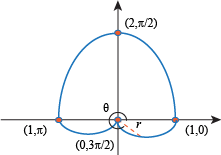
Again, we end with a heart shape that looks nothing like the graph

r = sin θ.

The moral is that adding constants does weird things to a polar graph.