Think you’ve got your head wrapped around Points, Vectors, and Functions? Put your knowledge to
the test. Good luck — the Stickman is counting on you!
Q. Consider the parameterization x = f(t), y = g(t) 0 ≤ t ≤ M where M is a constant greater than zero. Which of the following is the parameter?
x
y
M
t
Q. The point (4, 32) is on the graph of which set of parametric equations?
x = 2t, y = t2
x = t2, y = 2t
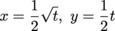
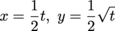
Q. Which of the following points is on the graph of the parametric equations

for t ≥ 0 ?
(-1, 0)
(-1, 2)
(5, 0)
(5, -1)
Q. Determine which picture best represents the graph of the parametric equations x = 2t2 and y = t2/2 for -4 ≤ t ≤ 4.


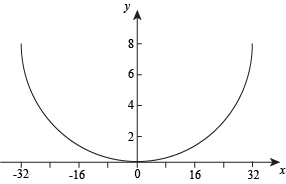
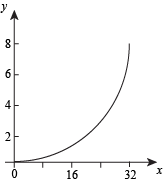
Q. Which of the following options are parametrizations of the unit circle?
I. x = cos t, y = sin t, 0≤ t ≤ 2π
II.
III.
IV.
(I) and (II)
(I) and (IV)
(II) and (III)
(II) and (IV)
Q. Determine which set of parametric equations traces the unit circle clockwise exactly once for 0≤ t ≤ 3π, starting at the point (1,0) when t = 0.
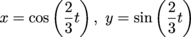


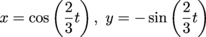
Q. All the following sets of parametric equations produce the same line, except for one. Which set of equations does not produce the same line as the others?
x = -6 + 6t, y = 2t
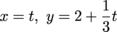
x = -6t, y = 2 – 2t
x = -6 + 2t, y = 2 + 6t
Q. Which parameterization produces the ray shown below?

x = -2 + 2t, y = -3 + 3t
x = 2 – 2t, y = 3 – 3t
x = -2 + 2t, y = -3 + 3t, t ≥ 0
x = 2 – 2t, y = 3 – 3t, t ≥ 0
Q. With the "usual" parameterization of the unit circle, x = cos t, y = sin t, which bounds on t are needed to trace exactly half the circle?
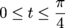
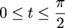
0 ≤ t ≤ π
0 ≤ t ≤ 2π
Q. Parameterize the line segment between the points (2, 4) and (5, 8).
x = 2 + 5t, y = 4 + 8t, 0 ≤ t ≤ 1
x = 5 – 2t, y = 8 – 4t, 0 ≤ t ≤ 1
x = 5 – 3t, y = 8 + 4t, 0 ≤ t ≤ 1
x = 2 + 3t, y = 4 + 4t, 0 ≤ t ≤ 1


