Increasing or Decreasing or... - At A Glance

What does it mean for something to be increasing or decreasing?

Albert is an industrious fellow. He decides to start his own lawn mowing business. When Albert gets paid for mowing his neighbor's lawn, he increases the amount of cash in his wallet. And when Albert decides go buy a pack of cotton candy bubble gum as a reward for his labors, he decrease that cash cache while increasing the tasty goodness in his mouth.

We say a function y = f(x) is strictly increasing if y gets bigger as x moves to the right. A strictly increasing function could look like one of these (or like many other things):

In symbols, if a < b then f(a) < f(b).

Sometimes, functions in real life are more complicated. We only deal with these functions in pieces called intervals.

We say a function y = f(x) is strictly increasing on the interval I if a < b implies f(a) < f(b) for all a, b in the interval I.

Sample Problem

The function y = f(x) graphed below is increasing on the interval [x_1, x₂], but not on the whole real line:

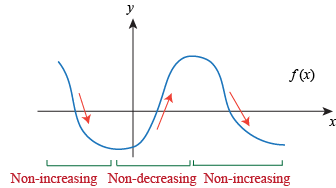
We say a function y = f(x) is nondecreasing if y doesn't get smaller as x moves to the right. The function doesn't have to get bigger, but it's not allowed to get smaller.

In symbols, if a < b then f(a) ≤ f(b).
A function can also be nondecreasing on a particular interval.

We can say a function is increasing at a point if its slope at that point is positive.

This is different than increasing over an interval.

We recommend not using the word increasing all by itself. We instead say strictly increasing or nondecreasing to be clear.

All these definitions are for functions that, more-or-less, increase. There are similar definitions for functions that, more-or-less, go down.

A function y = f(x) is strictly decreasing if the function is always getting smaller.

In symbols, if a < b then f(a) > f(b).

A function y = f(x) is nonincreasing if the function never gets bigger. The function might stay the same for a bit, but it never increases.

In symbols, if a < b then f(a) ≥ f(b).

Be Careful: strictly increasing and strictly decreasing aren't opposites. It's possible to have a function that's neither:

Similarly, nondecreasing and nonincreasing aren't opposites. It's possible to have a function that's neither:

There's one other term used when talking about the increasing-ness or decreasing-ness of functions: monotonic. No, we aren't talking about Ben Stein. A function is monotonic if it's going only one way. Therefore, it is either going up or going down.