Graph the function. Label all intercepts, critical points, and inflection points.

Answer

• Find dots.
The first thing to do is factor the numerator of f:
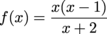
Now we can see that f is undefined with a vertical asymptote at x = -2, and has roots at x = 0 and x = 1.
The derivative of f is

This is zero when the numerator is zero, which occurs at (using the quadratic formula)

We have critical points at

The second derivative of f is

This is never 0, and is undefined at the same place f is undefined, so the function f has no inflection points.
Here are the dots:

• Find shapes.
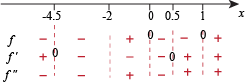
Here's the numberline:
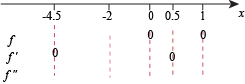
First, find the sign of the function  .
.
When x < -2 all quantities x, (x – 1), and (x + 2) are negative, so f is negative.
When -2 < x < 0 the quantities in the numerator are both negative and the denominator is positive, so f is positive.
When 0 < x < 1 the quantity x – 1 is negative, while x and x + 2 are positive, so f is negative.
When 1 < x all quantities x, x – 1, and x + 2 are positive, so f is positive.
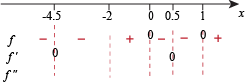
Next, the sign of  .
.
The denominator is always positive, so we don't need to worry about that. Since f '(0) is negative, the derivative f ' is negative in between the two critical points. Plugging in some other numbers, we can see what the derivative is doing outside the critical points.

and

The derivative f' is positive outside the critical points.
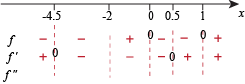
Finally, the sign of  . This one is negative when x < -2 and positive when x > -2.
. This one is negative when x < -2 and positive when x > -2.
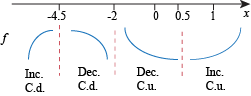
Using this, we find the shapes of f:
• Play connect-the-dots.
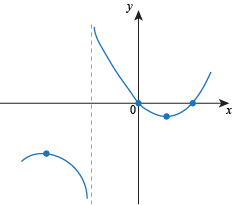
Here's the final picture.

 .
.


 , we have a critical point at (1, e).
, we have a critical point at (1, e).



 is positive when x is positive and negative when x is negative.
is positive when x is positive and negative when x is negative.
 is negative when x < 1 and positive when x > 1.
is negative when x < 1 and positive when x > 1.




 . The root x = 0 is also the y-intercept.
. The root x = 0 is also the y-intercept.
 The corresponding y values are
The corresponding y values are


 . f is also negative when x is positive and (x2 – 5) is negative, which occurs when
. f is also negative when x is positive and (x2 – 5) is negative, which occurs when  . f is positive everywhere else.
. f is positive everywhere else.
 and positive everywhere else.
and positive everywhere else.




























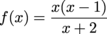






 .
.
 .
.


 . This one is negative when x < -2 and positive when x > -2.
. This one is negative when x < -2 and positive when x > -2.

