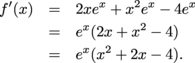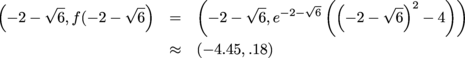Example 1
Find all intercepts, vertical asymptotes, critical points, and inflection points of the function. Label each point with its exact coordinates.
f (x) = ex
Example 2
Find all intercepts, vertical asymptotes, critical points, and inflection points of the function. Label each point with its exact coordinates.
f (x) = (x – 2)3
Example 3
Find all intercepts, vertical asymptotes, critical points, and inflection points of the function. Label each point with its exact coordinates.
f (x) = (1 – x)ex
Example 4
Find all intercepts, vertical asymptotes, critical points, and inflection points of the function. Label each point with its exact coordinates.
f (x) = x2ex – 4ex
Example 5
Find and graph all intercepts, vertical asymptotes, critical points, and inflection points of the function. Label each point with its exact coordinates.