Example 1
For the function, use the second derivative test (if possible) to determine if each critical point is a minimum, maximum, or neither. If the second derivative test can't be used, say so.

Example 2
For the function, use the second derivative test (if possible) to determine if each critical point is a minimum, maximum, or neither. If the second derivative test can't be used, say so.
f (x) = exsin x for 0 < x < 2π
Example 3
For the function, use the second derivative test (if possible) to determine if each critical point is a minimum, maximum, or neither. If the second derivative test can't be used, say so.
f (x) = x3 – 2x2 + x
Example 4
For the function, use the second derivative test (if possible) to determine if each critical point is a minimum, maximum, or neither. If the second derivative test can't be used, say so.

Example 5
For the function, use the second derivative test (if possible) to determine if each critical point is a minimum, maximum, or neither. If the second derivative test can't be used, say so.
f(x) = cos x on the interval

Example 6
Classify the extreme points of the function, using either the first or second derivative test. Explain why you chose to use the test you did.
f (x) = x4 – 32x
Example 7
Classify the extreme points of the function, using either the first or second derivative test. Explain why you chose to use the test you did.
f (x) = (x – 1)9
Example 8
Classify the extreme points of the function, using either the first or second derivative test. Explain why you chose the test that you did.

Example 9
Classify the extreme points of the function, using either the first or second derivative test. Explain why you chose to use the test you did.
f (x) = ex2 – 4x
Example 10
Classify the extreme points of the function, using either the first or second derivative test. Explain why you chose to use the test you did.







 and
and  , so these are our critical points. The second derivative is
, so these are our critical points. The second derivative is is negative, we know
is negative, we know 
 .
.  is positive, we know
is positive, we know
 .
.

 and x = 1. Now we find the (sign of) the second derivative at each critical point.
and x = 1. Now we find the (sign of) the second derivative at each critical point.



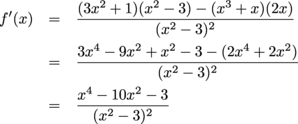
 , in which case f is also undefined, so we don't find any critical points that way. To find when f ' is zero we need to solve the equation
, in which case f is also undefined, so we don't find any critical points that way. To find when f ' is zero we need to solve the equation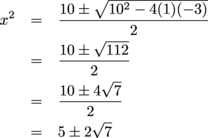
 is negative and x2 can't be negative, we know
is negative and x2 can't be negative, we know
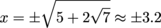


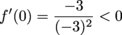
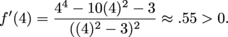
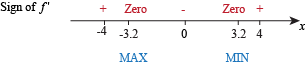
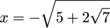
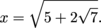



 . We can't use the second derivative test at x = 0 because f '(0) doesn't even exist. The first derivative test it is. Here's the numberline, and the values we need to plug into f':
. We can't use the second derivative test at x = 0 because f '(0) doesn't even exist. The first derivative test it is. Here's the numberline, and the values we need to plug into f':


