Example 1
For the function, use the First Derivative Test to determine if each critical point is a minimum, a maximum, or neither.
 for 0 < x
for 0 < x
Example 2
For the function, use the First Derivative Test to determine if each critical point is a minimum, a maximum, or neither.
f (x) = -x3 + 3x2 – 3x
Example 3
For the function, use the First Derivative Test to determine if each critical point is a minimum, a maximum, or neither.

Example 4
For the function, use the First Derivative Test to determine if each critical point is a minimum, a maximum, or neither.

Example 5
For the function, use the First Derivative Test to determine if each critical point is a minimum, a maximum, or neither.
f (x) = ex







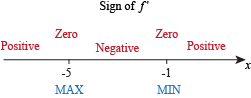




 and positive to the right of x =
and positive to the right of x =  , f has a minimum at x =
, f has a minimum at x =  . Since f ' is positive to left of x =
. Since f ' is positive to left of x =  and negative to the right, f has a maximum at x =
and negative to the right, f has a maximum at x =  .
.