Think you’ve got your head wrapped around Second Derivatives and Beyond? Put your knowledge to
the test. Good luck — the Stickman is counting on you!
Q. A critical point of a function f is a point at which
f ' is zero
f ' is zero or undefined
f " is zero or undefined
f " changes sign
Q. Which of the following statements about the function
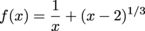is true?
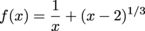is true? The function f has no critical points.
x = 0 is the only critical point of f.
x = 2 is the only critical point of f.
x = 0 and x = 2 are both critical points of f.
Q. An inflection point of a function f is a point at which
f ' is zero
f ' is zero or undefined
f " is zero or undefined
f " changes sign
Q. Which of the following statements about the function f(x) = 4(x – 1) – 8 is true?
The function f has no inflection points.
x = 1 is the only inflection point of f.
x = 3 is the only inflection point of f.
x = 1 and x = 3 are both inflection points of f.
Q. A maximum value of a function f is
a y-value that is bigger than every other value of f.
a y-value that is bigger than every nearby value of f.
an x-value at which f is bigger than anywhere else.
an x-value at which f is bigger than anywhere nearby.
Q. How many minima does the function f(x) = -5cos x have?
none
one
finitely many, but more than one
infinitely many
Q. x = c is a critical point of the function f. The sign of f ' is negative to the left of c and positive to the right of c. Which of the following statements is true?
x = c is a maximum value of f.
A maximum value of f occurs at x = c.
x = c is a minimum value of f.
A minimum value of f occurs at x = c.
Q. x = c is a critical point of the function f. If f "(c) = 0, which of the following statements is true?
The second derivative test says that a minimum value of f occurs at x = c.
The second derivative test says that a maximum value of f occurs at x = c.
The second derivative test says that neither a minimum nor a maximum value of f occurs at x = c.
The second derivative test says nothing at all about what occurs at x = c.
Q. If the derivative of a function f is f '(x) = (x – 2)(x + 5), which of the following statements is true?
f has a minimum at x = 2 and a maximum at x = -5.
f has a maximum at x = 2 and a minimum at x = -5.
f has minima at both x = 2 and x = 5
f has maxima at both x = 2 and x = 5
Q. The derivative of the function f is
f '(x) = 4x + 8
Which of the following statements is true?
f has a minimum at x = 2.
f has a maximum at x = 2.
f has a minimum at x = -2.
f has a maximum at x = -2.