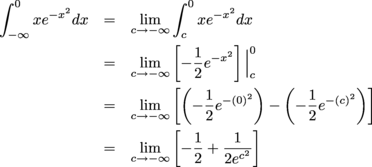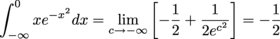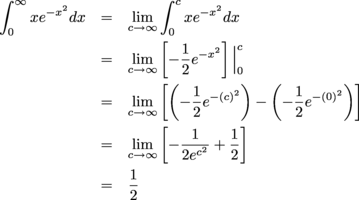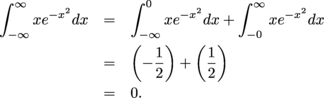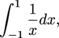Badly Behaved Limits Examples

Determine whether

converges or diverges, and find its value if it converges.

We've sort of done this problem already. We're pretty sure that this integral converges, and that it converges to 1. Let's argue the case a little more carefully. By the definition of this type of improper integral, we know that

We know how to work out this integral, so we do - remembering to keep the limit notation along for the ride.

We've worked out the integral inside the limit, so it's time to figure out the limit. As b approaches ∞, the term  approaches 0. So

The integral

converges to 1.

Remember to include the limit. If you leave a limit out, you get something like

which doesn't make any sense. The left-hand side is a limit, which is either a number or doesn't exist at all. The right-hand side is just an algebraic expression with the letter b in it.

Determine if

converges or diverges. Find its value if it converges.

We do pretty much the same stuff we did for the last problem.

As b approaches -∞ the quantity  approaches 0, so we have

The function  is even, so this answer makes sense. We're saying the area between  and the x-axis on (-∞,-1) is the same as the area between  and the x-axis on (1,∞).

Determine if

converges or diverges. If the integral converges, find its value.

We write the improper integral as the limit it really is, work out the definite integral, then work out the limit.

As b approaches ∞, the quantity ln b also approaches ∞. That means this limit doesn't exist, so we say the integral

diverges. That means this region has infinite area.

Determine if

converges or diverges. Find its value if it converges.

We need to split up the integral so we can deal with one badly-behaved limit of integration at a time. Let's split the integral at 0, since that's a nice easy number.

Now we need to work out each of the simpler improper integrals (unless the first one diverges, in which case we'll be done!).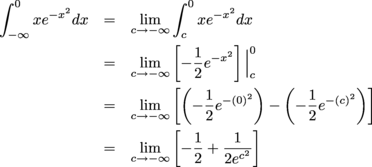

Since e^c2 approaches ∞ as c approaches -∞, the limit converges and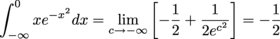

The other improper integral is similar.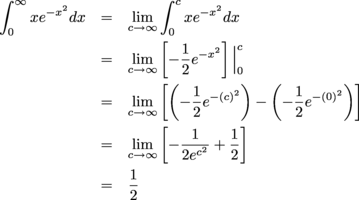

Putting our results together,

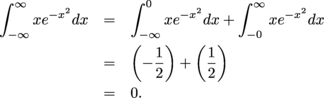
The original integral converges to 0. This makes sense, because in the graph it really looks like the total weighted area between the function and the x-axis should be 0.

Yes, that was a lot of work to get 0 as an answer. Sometimes that's how it goes. And yes, it does make a lot of sense when you look at the graph that you would get zero for that integral. Alas, not all odd functions are that nice. The integral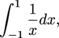

for example, doesn't exist.