Integration by Parts: Indefinite Integrals Examples

Use integration by parts to find .

We'll do this example twice, once with each sort of notation.

Using prime notation, take

u = x

v' = e^x

Then u' = 1 and v = e^x. We plug all this stuff into the formula:

Since the integral of e^x is e^x + C, we have

We write + C instead of – C since either way we're describing the same family of functions.

Using fraction notation, take

u = x

dv = e^xdx

Then du = dx and v = e^x. We plug all this stuff into the formula:

Since the integral of e^x is e^x + C, we have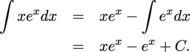

Much to everyone's dismay, there's no set of absolute rules for determining which function should be u. There are some guidelines, though. The whole point of integration by parts is that if you don't know how to integrate

,

you can apply the integration-by-parts formula to get the expression

and hopefully this second integral will be easier to integrate than the original integral.

If you pick u and v' incorrectly the first time, you'll probably realize it soon.

Sample Problem

If we try to integrate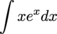

by parts, and we choose

u = e^x

v' = x

then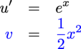

Putting everything into the formula, we get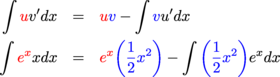

The new integral is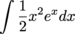

which is worse than the original. This means we didn't pick u correctly!

Thankfully, if we chose poorly the first time, all it means is we have to start over.

When picking u and v', keep these guidelines in mind:

• u ' should be simpler than u (or at least not worse!)
    
• v should be simpler than v' (or at least not worse!)
    
• You need to be able to find v from v'
    
• The new integral should be easier to integrate than the original integral.