We want to write 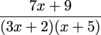
as a sum of fractions 
We don't know what A and B are, but we know if we add 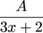
and 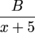
we'll get 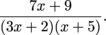
Writing this as an equation, we want to find A and B so that 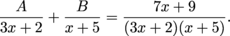
We know how to add rational functions, so we know that 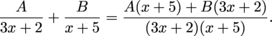
We want this sum to equal the original fraction, so we want 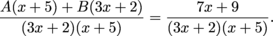
The denominators of these fractions are the same, so the fractions are the same if their numerators are equal. This means we need to find A and B so that A(x + 5) + B(3x + 2) = 7x + 9. There's an easy way and a harder way to do this. The harder way is to multiply out the left-hand side, find that (A + 3B)x + (5A + 2B) = 7x + 9, and solve the system of equations A + 3B = 7 5A + 2B = 9. We don't want to do that, because it's too much work. Instead, we'll carefully choose values of x so that we're solving for only one of A or B at a time. If we take x = -5, for example, then we don't have to worry about A. A(x + 5) + B(3x + 2) = 7x + 9 A((-5) + 5) + B(3(-5) + 2) = 7(-5) + 9 A(0) + B(-13) = -26 B = 2. Now that we know B = 2, we can use any value of x we like to solve for A. Let's take x = 0. A(x + 5) + B(3x + 2) = 7x + 9 A((0) + 5) + (2)(3(0) + 2) = 7(0) + 9 5A + 4 = 9 5A = 5 A = 1. Now we know that A = 1 and B = 2, so we've successfully decomposed the original fraction: 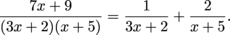
As long as the denominator of the original function factors into distinct linear factors, there will be one partial fraction for each factor of the denominator. For the time being
we'll be nice and give you the original function with the denominator already factored. |