Since the integrand is of the form  where p > 1 and the interval of integration is [0,1], this integral diverges. Piece of cake, right? The hard part is remembering when you want p > 1 and when you want p < 1. There's a great trick for that: you can get by with remembering just one integral. The integral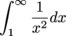 
converges because  gets close to the x-axis quickly as x approaches ∞. If you can remember this, then you can remember that 
because you have an example where p = 2 is greater than 1 and the integral converges. That means 
We switch between p > 1 and p < 1 when we change the interval of integration from [1,∞) to [0,1], so this means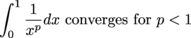 
and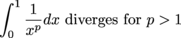 
That's the whole p-test, and all we had to remember was that the integral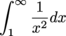 
converges. We recommend taking your time when using the p-test. It can be easy to get mixed up, even if you know what you're doing! | 