If a is a constant and the series

converges, what is the value of a?
Answer
If every term is a, then the partial sums are
S1 = a
S2 = 2a
S3 = 3a
...
Sn = na
If a ≠ 0, then the values na grow without bound as n gets large, so
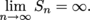
This means if a ≠ 0 the series diverges. The only way the series can converge is if a = 0.
We mentioned earlier that any finite series can be thought of as an infinite series by sticking on infinitely many copies of 0 at the end.
Suppose we start cleaning out the guts of a giant pumpkin so we can carve a hideous face into it. Every day we record the hours we spend cleaning it out. It's so big that it looks like we could never finish it, but, lo and behold, one day it's cleaned out. Every day after that the number of hours we spend pumpkin prepping is 0, and our total time gourd gutting stays the same.
If we do the same for any finite series, we get some finite number L. After this point, all further partial sums will also be equal to L. Since the partial sums converge to L, the original series converges to L. Any finite series converges. We prefer our pumpkin proof over our more monotonous demonstration.



