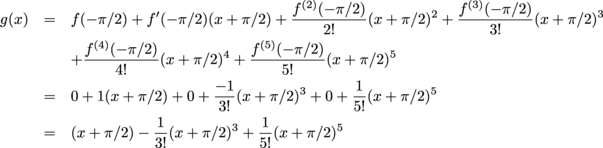Example 1
Let f(x) = sin(x). By taking derivatives, find a function g(x) of the form g(x) = a + bx + cx2 that has the same value, slope, and second derivative as f when x = 0.
Graph f and g on the same axes.
Example 2
Find the 5th-degree Taylor polynomial centered at 0 for cos x.
Example 3
What is the Maclaurin series for f(x) = cos x (a.k.a. the Taylor series for f(x) = cos x near x = 0) ?
Example 4
What is the nth term, or general term, of the Taylor series for f(x) = ex near x = 0?
Example 5
(a) Find the Taylor series for  .
.
(b) Why does your answer make sense?
Example 6
Which is the Taylor series for the function f(x) near x = 5 ?
(A) 
(B) 
(C) 
(D) 
Example 7
Find the Taylor series for the function f(x) = ln x at a = 1.
Example 8
Which of the following functions could be the second degree Taylor polynomial for the function f(x) near -π/2?

Example 9
Find the 5th degree Taylor polynomial for the function f(x) = cos x at  .
.
Example 10
Find the first four nonzero terms of the Taylor series for f(x) = x1/3 centered at x = 1.






 .
. before. Where?
before. Where?





 is the sum of the infinite geometric series with ratio x, where |x| < 1.We just found that the Taylor series for
is the sum of the infinite geometric series with ratio x, where |x| < 1.We just found that the Taylor series for  near zero is
near zero is


 term.
term. . Since
. Since  , we don't even have to think to evaluate half of these derivatives.
, we don't even have to think to evaluate half of these derivatives.