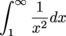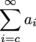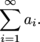The Integral Test Examples

Does the constant series

where a > 0, converge or diverge?

We already know the constant series diverges if a ≠ 0.

However, looking at the graph can help the intuition. Here's a 2-D graph of the constant series:

Putting all the little rectangles together creates an infinitely long rectangle of height a > 0. The area of such a rectangle must be infinite. Since the sum of the constant series is the area of that rectangle,

is infinite - in other words, the series diverges.

At long last, we can give a proof that the harmonic series diverges (even though its terms converge to 0).

Does the harmonic series

converge or diverge?

Look at the visualization of the harmonic series where we use left-hand sums.

Draw the curve

through this picture. Since all the dots corresponding to terms of the series have coordinates of the form

the curve  passes through all the dots.

A while ago we found that

diverges, which means the area it represents is infinite.

Since the rectangles cover even more area, the area covered by the rectangles must be infinite also.

The sum of the harmonic series

is the area covered by the rectangles, which is infinite, so the harmonic series diverges.

Another way to look at this example is that the rectangles we get by visualizing the harmonic series give a left-hand sum approximating the integral

Since the function  is decreasing, a left-hand sum is an overestimate . An overestimate of an infinite value is infinite, so

is infinite. The series diverges.

Does the series

converge or diverge?

Since the function  is non-negative and decreasing on [1,∞) we can use the integral test. The integral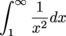

converges by the p-test . The integral test says that the series must also converge. In pictures, the area described by the integral is finite. Since the rectangles that correspond to the terms of the series cover a smaller area, this smaller area must also be finite. This means the series converges.

There's something a tiny bit tricky going on in this example. The rectangles that give the underestimate of

don't correspond exactly to the series

They correspond to the series

because of how we're drawing the rectangles:

This is one of those times when limits of summation don't matter . The first term a₁ of the series is a finite number, so if

converges then so does the original series

This is why we have the part about c in the integral test. Since limits of summation don't matter for the convergence/divergence of a series, if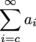

converges, then so does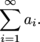

We can use any starting index we like, so long as all the terms are defined.

This means the convergence/divergence of the infinite series

a₁ + ... + a_{c – 1 +} a_{c + ...}

is determined by the converge/divergence of its tail a_c + a_{c + 1} + .... If the tail converges, the whole series converges. If the tail diverges, the series diverges.

This means if the starting index of the series isn't a good choice for c, we can pick a different value. We can pick any whole number value of c for which f is non-negative and decreasing on [c,∞).

If possible, use the integral test to determine whether the series

converges or diverges.

The function

doesn't behave exactly as we want: it isn't non-negative and decreasing from 1 onwards. However, if we zoom in on the graph it appears

that f(x) is non-negative and decreasing from x = 6 onwards:

If we wanted to be careful, we could show that f' is indeed non-negative for all x > 6. Since f(x) is non-negative and decreasing on [6,∞) we can use the integral test with the integral

Using integration by parts we get

It doesn't matter what this works out to exactly. The important thing is that the terms -ce^-c, -e^-c, and 5e^-c approach 0 as c approaches ∞, so the limit works out to a finite value. This means the integral converges, so by the integral test

converges. Since the starting index of summation doesn't matter,

also converges.

If possible, use the integral test to determine whether the series

Σ sin n

converges or diverges.

We can't use the integral test here. There is no whole number value of c for which the function f(x) = sin x is non-negative and decreasing on [c,∞). However, since

doesn't exist, we can use the divergence test to say that this series diverges.