The first term, a1 = 1, is the area of a rectangle with height 1 and width 1. We are using rectangles now because we ate all of the grilled cheese sandwiches. Put this rectangle on the interval [1, 2]: 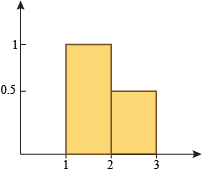
The next term,  , is the area of a rectangle with height , is the area of a rectangle with height  and width 1. Draw this rectangle on the interval [2, 3]: and width 1. Draw this rectangle on the interval [2, 3]: 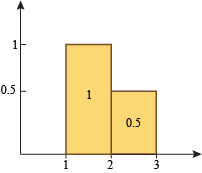
Keep going. The area of the nth rectangle is 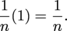
Draw this rectangle on the interval [n, n + 1]: 
If we add up the areas of all the rectangles, we get the harmonic series: 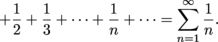
The sum of the series is the total area covered by the rectangles. We're not claiming the area is finite. In fact, in this case it isn't, as we'll show soon. However, it still makes sense to think of the area covered by the rectangles as the sum of the series. In the previous example we drew the rectangle for an on the interval [n, n + 1]. This image looks surprisingly familiar to the left-hand sum of an integral. This is because the value of the series at n (the left endpoint of the interval) determines the height of the rectangle. Alternately, we could draw the series by putting the rectangle for an on the interval [n – 1, n]. In this case we get a right-hand sum. The value of the series at n (the right endpoint of the interval) determines the height of the rectangle. |