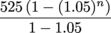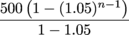Think you’ve got your head wrapped around Series? Put your knowledge to
the test. Good luck — the Stickman is counting on you!
Q. The series 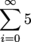 is
is
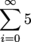 is
is an arithmetic series
a geometric series
neither an arithmetic nor a geometric series
both an arithmetic and a geometric series
Q. 0.5, 0.25, 0.125, ... is a(n)
arithmetic sequence
arithmetic series
geometric sequence
geometric series
Q. The nth partial sum of a geometric series is
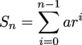
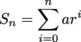
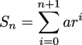
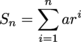
Q. Find 

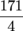
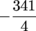

The sum doesn't exist because |r| > 1.
Q. The sum of the infinite geometric series
 ,
,
where a ≠ 0, is
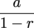 for all r
for all r 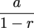 for |r| < 1, undefined otherwise
for |r| < 1, undefined otherwise 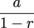 for |r| ≤ 1, undefined otherwise
for |r| ≤ 1, undefined otherwise  for -1 ≤ r < 1, undefined otherwise
for -1 ≤ r < 1, undefined otherwise Q. Find  .
.
 .
. 


1
Q. Let a be a constant. If the series  converges then we must have
converges then we must have
 converges then we must have
converges then we must have a = 0
|a| < 1
0 < a < 1
-1 < a < 0
Q. We can write the decimal 0.421421421... as a rational number using an infinite geometric series with r =

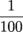

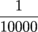
Q. Which of the following expressions could be considered to be in "closed form"?
(I) a + ar + ar2 + ... + arn – 1
(II) 
(III) 
(II) only
(III) only
(I) and (II)
(II) and (III)
Q. Every year Leopold puts $500 into his bank account and then earns 5% interest on the total contents of his account. At the end of the first year, his bank account contains $525. How many dollars are in his bank account at the end of the nth year?