Word Problems Examples

On the first day of February Liam feeds one penny to his piggy bank. On each subsequent day he feeds his piggy bank twice as many pennies as he did the day before.

(a) How much money is in Liam's piggy bank at the end of the first week of February?

(b) How much money is in Liam's piggy bank at the end of February? Assume it's not a leap year.

This story describes a geometric series. On the nth day of February, Liam feeds his piggy bank a_n pennies. We start with

a = a₁ = 1.

Since the number of pennies is doubled each day, the ratio is r = 2.

(a) After one week of feeding, Liam's piggy bank has been fed n = 7 times. It contains a total of

(b) By the end of February, Liam's piggy bank has been fed n = 28 times. It contains

pennies or $ 2,684,354.55. If only we could really save that much money in a month by feeding pennies to piggy banks.

On January 1st Kendra puts $100 into her bank account. On the first of each month after that she deposits an additional $100. Unfortunately, during the middle of the month she always has to withdraw 75% of her money to pay bills.

(a) How much money is in Kendra's account after her March deposit?

(b) How much money is in Kendra's account after the nth deposit? Write your answer in closed form.

The timeline is going to be useful here. Different things are happening to the bank account at different times of the month, and the questions are asking about the state of the bank account at different times of the month.

To start, here's what happens in January: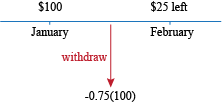

If Kendra withdraws 75% of her money, that means 25% is left, so she has

(0.25)(100) = $25

in her account at the end of January.

(a) Extend the timeline. At the end of January, Kendra has $25. At the beginning of February she deposits another $100, so she now has $125 in the bank. In the middle of February she withdraws 75% of her money, leaving 25% of that $125 in the bank. At the end of February her bank account contains

0.25(125) = 31.25 dollars.

At the beginning of March she deposits $100, so now her account holds

100 + 31.25 = $131.25.

(b) Let M_n be the amount Kendra has in the bank after her nth deposit. Her nth deposit is $100, and she still has 25% of M_{n – 1} left over from the previous month. This means

M_n = 100 + 0.25M_{n – 1}.

It's not practical to use this formula for direct calculations. If we wanted to calculate, say, M₂₀, we would first have to calculate M₁ through M₁₉.

However, since this formula tells us how to get one value of M_n from the previous value, we can use the formula to find a more useful way of expressing M_n.

Following the hint of not over-simplifying, use the useful trick of writing M_n as a series, and look at some values of M_n where n is small: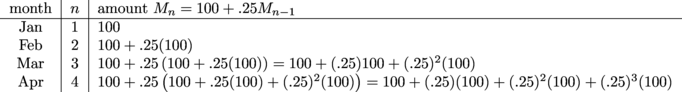

Each M_n is the sum of a finite geometric series. The exponents in the finite series go from 0 to (n – 1).

The value of M_n is the sum of a finite geometric series with a = 100, r = 0.25, and n terms (since the exponents go from 0 to (n – 1)). Using the formula for the sum of a finite geometric series,

Try these questions, which also ask about the same situation as the example we just saw.