A Match Made in Math-Heaven
If series are peanut butter, then integrals are jelly. You could put one or the other on a couple slices of bread and have a satisfying sandwich. You could also put them both together and have a tantalizing treat in your lunch box.
The two go together so nicely for a reason. Although they're very different, they have some nice properties that are very similar. It's no coincidence. Remember that an integral is defined in terms of a limit that the left hand sum (LHS) and the right hand sum (RHS) are the same. An infinite number of intervals is usually used in this limit, so these sums look just like infinite series.
There is one difference. To use these properties, we have to know already that the series converges. After all, peanut butter doesn't taste like jelly. They just go well together.
Assuming the series
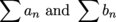
both converge, then
If 
converges and c is a constant, then
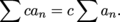
and 
converge, we can break up the series:
